In my previous article, Beating The S&P 500 With An Apple-Tilted ETF Portfolio. I used the website etfreplay.com to construct an ETF portfolio that outperformed the S&P 500 over 1 year, 2 year, and 3 year periods with lower volatility than the S&P 500 and with exposure to Apple (NASDAQ:AAPL ). The portfolio I created had a 20% allocation to a bond ETF, the Vanguard Intermediate-Term Bond ETF (NYSEARCA:BIV ). Including bonds in a portfolio is an essential part of diversification. Without getting into the complex bond terminology of duration, coupon payments, and the like (which sometimes even confuses myself), the use of bonds in a portfolio can be clarified as follows: bonds reduce the volatility of a portfolio while providing a small, steady stream of income. In doing so, bonds increase a portfolio’s Sharpe ratio (or reward-to-risk ratio).

Taking into consideration that individual investors don’t have the capital required to buy bonds directly, most investors probably get their exposure to bonds through mutual funds or ETFs. In the world of ETFs, a simple solution can be found in the form of a total bond market ETF or ETFs that specialize in a particular time to maturity (short, intermediate, or long-term). Even further diversification within bonds is possible with a high-yield corporate or junk bond ETF.

Two very popular total bond market ETFs are the iShares Barclays Aggregate Bond Fund (NYSEARCA:AGG ) and the Vanguard Total Bond Market ETF (NYSEARCA:BND ). Both track the same index, the Barclays Capital U.S. Aggregate Index. The index has 7,854 bonds and is very hard to replicate. The main difference between BND and AGG (besides net expense ratio with BND at 0.11 and AGG at 0.22; BND is also much bigger) is that BND has a higher number of holdings than AGG. In other words, BND will be able to track the index better.

Indeed, I have seen many asset allocation plans in investing magazines that use either AGG or BND as the core bond holding in a portfolio of ETFs. Various pluses and minuses accompany the use of these ETFs. A distinct positive is that one can own just one ETF and have exposure to a wide variety of bonds. However, such an ETF does not offer much diversification with respect to the maturities of bonds.

While visiting Vanguard’s website (the fund family of BND), I stumbled across the following the following table relating to BND’s holdings:

One can clearly see that this ETF’s holdings are concentrated towards short to medium-term bonds. If short term bonds are classified as having maturities of 1-3 years, and medium term bonds as having maturities of 3-7 years, then BND’s holdings in short to medium term bonds are around 70.5% (2.5% + 24.8% + 27.7% + 0.5 x 30.9% or 15.45%).

As part of this article, I decided to run 3-year backtests on portfolios using hypothetical stock/bond ratios. I did these backtests because I wanted to get an overall picture of how bond ETFs affect a portfolio’s Sharpe ratio. I then created graphs to visualize the data. In each portfolio, the percentage allocation to stocks decreases from 100 to 0 in increments of 10 (and likewise, the allocation to bonds increases from 0 to 100, also in increments of 10). I used a single ETF, the Vanguard Total Stock Market ETF (NYSEARCA:VTI ) to act as a proxy for my stock holdings. This ETF serves well as it has exposure to medium and small-cap stocks, something that an ETF like the SPDR S&P 500 ETF (NYSEARCA:SPY ) — an ETF that tracks the S&P 500 index — lacks since it only consists of large-cap stocks.

First, I started out with a graph of a mix of VTI and BND as follows:

One can see that the Sharpe ratio increases exponentially until a 10/90 stock/bond allocation is reached. The Sharpe ratio at this allocation is 2.2. This acts as an inflection point since the next Sharpe ratio at the 0/100 allocation is lower. At 1.49, this Sharpe ratio is actually just about the same as the Sharpe ratio at the 30/70 allocation.

Now, earlier I wrote that investors have the choice of buying a total market bond ETF or specializing and buying an ETF with a certain maturity (either short, medium, or long-term). It just so happens that short, medium, and long-term bonds each have their own characteristics. For this article, I chose three different bond ETFs to understand how each impacts the Sharpe ratio in a portfolio. The Bond ETFs are as follows:

• The Vanguard Short-Term Bond ETF (NYSEARCA:BSV ) for short-term bonds, with maturities of 2-3 years and a net expense ratio of 0.11.
• The Vanguard Intermediate-Term Bond ETF for intermediate-term bonds, with maturities of 6-7 years and a net expense ratio of 0.11.
• And finally, the Vanguard Long-Term Bond ETF (NYSEARCA:BLV ) for long-term bonds, with maturities of 12+ years and also with a net expense ratio of 0.11.

I made a single graph for all of the stock/bond allocations using the ETFs above, each line represents the use of a different ETF in combination with VTI:

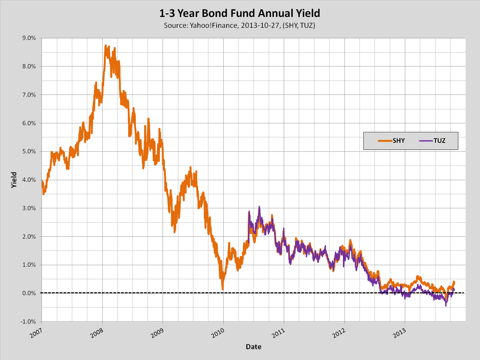
This graph might look confusing at first glance, but there’s really only three things to make note of. While all portfolios start at the same Sharpe ratio, the portfolio with long-term bonds has a Sharpe ratio that increases at a faster rate than the other two portfolios before it reaches its inflection point. Then, the portfolio with medium-term bonds has a Sharpe ratio that increases at a rate that is between the other two before it reaches its inflection point. Finally, the portfolio with short-term bonds has a Sharpe ratio that increases at the slowest rate before it too reaches its own inflection point.

Analysis and Findings

Now, here is some analysis. By comparing the two graphs above, one can see that the Sharpe ratios of the portfolios with medium and long term bond ETFs are each greater than the portfolio with the total bond market ETF. This means that, theoretically, an investor can increase performance if her or she diversifies within bond holdings by buying equal amounts of short-term, medium-term, and long-term ETFs. Doing this would take the place of buying a single total market bond ETF. The allocation to medium and long-term bond ETFs should boost performance beyond that which can be attained by simply by buying a total bond market ETF.

To test this theory I graphed the Sharpe ratios of a portfolio that uses VTI for stock holdings and then an equal weight split among BSV, BIV, and BLV for bond holdings. Interestingly, just adding together the Sharpe ratios of the second graph above wouldn’t lead to much insight as the Sharpe ratio of a portfolio isn’t equal to the sum of its parts (this goes in hand with the fact that the standard deviation of a portfolio isn’t equal to the sum of the standard deviations of its components). The results are as follows:

When compared to the first graph, one can see that the Sharpe ratios of such a portfolio — one that uses an equal-weighted split among short, medium, and long-term bond ETFs in its allocation to bonds — are greater than those of a portfolio that uses a total bond market ETF at almost every stock/bond allocation mix; that is, until one reaches a mix of 10% stocks and 90% bonds. The general rule of thumb is that investors should use a formula of 100 minus his or her age as the percent allocation to bonds. So, for investors 90+ years of age (and those with less than 10% in stocks), it would be wiser to use a total bond market ETF over an equal-weighted split. I would venture to guess that most investors would have less than a 40% allocation to bonds and would benefit from an equal-weighted bond ETF plan.

Of course, this article can’t be read without some healthy criticism. Among its faults is that it heavily relies on the use of backtests — and over a time period that actually favors long and medium-term bonds. As interest rates rise, the bond prices of these maturities will fall, which in turn will affect the performance of a portfolio with an equal-weighted short, medium, and long-term bond ETF allocation. The Sharpe ratio of such a portfolio would decrease as its return decreases and its volatility increases. On the other hand, I think the overall Sharpe ratio would depend on the characteristics of the underlying bond ETFs on a relative basis. For example, as long-term bonds decrease in price, a portfolio of VTI and BLV would then have a lower Sharpe ratio at each Stock/Bond allocation mix and an inflection point that would be pushed towards the start of the graph. I’m doubtful that any inflection point would be pushed to a point before a 60/40 minimum stock and maximum bond combination, of which most investors probably adhere to (in other words, most investors probably have more than 60% in stocks and less than 40% in bonds). The same thing would hold true for a portfolio of VTI and BIV (and also possibly for one of VTI and BSV in the case of short-term bonds). The end result is that while its true that these ETFs would then lower the overall Sharpe ratio in an equal-weighted strategy for bonds, I’m positive that the performance of such a portfolio would still be greater than one that uses a single total bond market ETF.

Two very important, additional points can be made. To achieve similar performance with the bond portion of a portfolio being equal weighted among maturities, the investing environment must be similar to conditions that were encountered in the backtests. In short, an investor must implicitly assume that the stock market will continue to climb in the same manner that VTI did over the past three years. VTI’s percentage increase over the past three years was about 21%, which is a compounded annual growth rate of about 2.8%. Considering that the average annual return of the stock market clocks in at around 6-7%, going forward it should theoretically be very feasible to exceed VTI’s 2.8% over the past three years. Indeed, over the entire history of the stock market, stocks have gone up most of the time (I think the actual number is around 75-80% of the time)! The other point worth noting is that the Fed funds rate will more than likely start rising within the near future. The Fed funds rate has been at record lows over the past three years. So how can I say that similar performance can be observed going forward? The answer is that I backtested from January 1 2010 to May 1 2010, a 5 month window of time when the effective Fed funds rate gradually rose. What I found was that the portfolio with equal weighted bond ETFs outperformed the one with just a total market bond ETF at almost every step (except for the extreme 10/90 and 0/100 stock and bond allocations). If the two methods both had the same performance attributable to stocks, then the extra performance must come from being equal weighted in bond ETFs. While this evidence doesn’t make the theory in this article fool-proof, it certainly seems to support it.

After thinking about things for a while, I developed an alternative way of explaining the outperformance: by using equal-weighted bond ETFs, no active bets are being placed on any one bond maturity. Thus, any poorly performing ETF will be balanced out by the other two (and the opposite is true as well in the case of an ETF that performs very strongly). The main point I am trying to convey is that extremes will be smoothed out in the long term, leading to a slow but steady increase in performance over time.

Conclusion

Bonds are an important part of diversification within an investor’s portfolio. They can be confusing to the average investor, but they are readily accessible through mutual funds and ETFs. As shown in this article, a bond ETF’s time to maturity and its allocation are related to how that ETF behaves in a portfolio and affects it. An investor who uses a total bond market ETF for his or her exposure to bonds should consider switching to an allocation that uses equal weighted bond ETFs for exposure in this asset class. Such an allocation can be expected to lead to superior performance. It should then be relatively easy to re-balance within bonds, since the ETFs will be held in equal proportions (no hard math or algorithms needed!). As an added bonus, implementing such an allocation with the Vanguard short, medium, and long-term bond ETFs mentioned in this article will not have a higher net expense ratio than using Vanguard’s total bond market ETF (all 4 have the same 0.11 net expense ratio!). Such an allocation is also cheaper than AGG’s net expense ratio of 0.22. Alternatively, if an investor does not wish to sell shares of BND or AGG already owned, he or she can get additional exposure to long-term bonds buy putting about 20% of an equivalent cash value of BND or AGG into a long-term bond ETF such as BLV (since 30% of BND and AGG are in long-term bonds, this will then increase the weighting in long-term bonds to 50%). Bonds don’t have to be difficult with this simple plan to follow.

Disclosure: I have no positions in any stocks mentioned, and no plans to initiate any positions within the next 72 hours.

Conduct in-depth research on BND and 1,600+ other ETFs with SA’s ETF Hub