Margins Multiples and the Iron Law of Valuation
Post on: 15 Июль, 2015 No Comment
Midweek Matters
Dan Steinhart. Managing Editor, The Casey Report
Garbage In, Garbage Out.
This well-worn phrase describes how feeding flawed data into a computer guarantees that it will spit out flawed results.
It applies equally to human endeavors: if you attempt to answer a question using bad data, your answer will be wrong, no matter how rigorous your analysis.
Thats why the ability to separate important information from garbage is one of the most useful skills an investor can possess. With statistics like these constantly bombarding us
Short Facebookits price-to-book ratio is 10.8!
Load up on Exxon sharesits price/earnings ratio is just 13.3!
Dont even think about buying Blackberryits short interest is over 20.4%!
we need to know which ones to pay attention to, and which ones to ignore.
Todays guest author, fund manager, and frequent contributor to this missive, John Hussman. is the king of making such distinctions. He often scolds pundits in his weekly market comment series for citing statistics that make for good sound bites, but dont correlate at all to what theyre trying to prove.
In what follows, John reveals a few lesser-known valuation metrics that actually do have proven track records for predicting where the stock market is going. Read on to learn about them, and what they say about how the stock market will perform over the next several years.
Dan Steinhart
Managing Editor of The Casey Report
Margins, Multiples, and the Iron Law of Valuation
John Hussman. President, Hussman Investment Trust
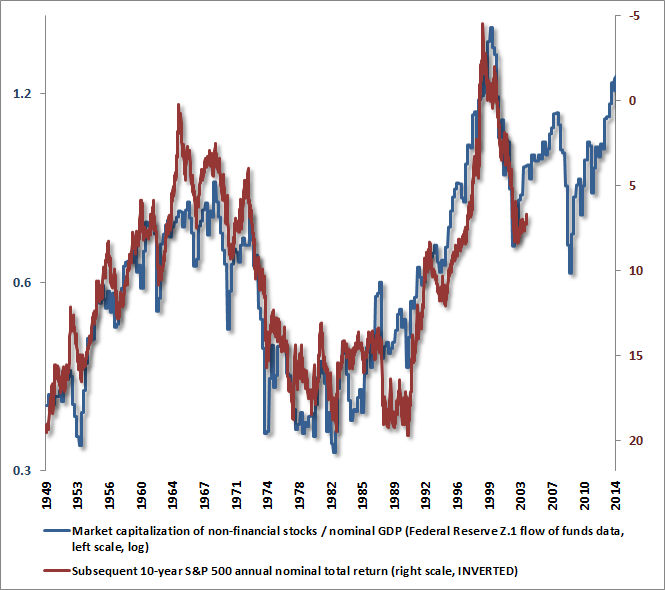
The equity market remains valued at nearly double its historical norms on reliable measures of valuation (though numerous unreliable alternatives can be sought if one seeks comfort rather than reliability). The same measures that indicated that the S&P 500 was priced in 2009 to achieve 10-14% annual total returns over the next decade presently indicate estimated 10-year nominal total returns of only about 2.7% annually. Thats up from about 2.3% annually last week, which is about the impact that a 4% market decline would be expected to have on 10-year expected returns.
I should note that sentiment remains wildly bullish (55% bulls to 19% bears, record margin debt, heavy IPO issuance, record covenant lite debt issuance), and fear as measured by option volatilities is still quite contained, but tail risk as measured by option skew remains elevated. In all, the recent pullback is nowhere near the scale that should be considered material. Whats material is the extent of present market overvaluation, and the continuing breakdown in market internals were observing. Remembermost market tops are not a moment but a process. Plunges and spikes of several percent in either direction are typically forgettable and irrelevant in the context of the fluctuations that occur over the complete cycle.
The Iron Law of Valuation is that every security is a claim on an expected stream of future cash flows, and given that expected stream of future cash flows, the current price of the security moves opposite to the expected future return on that security. Particularly at market peaks, investors seem to believe that regardless of the extent of the preceding advance, future returns remain entirely unaffected. The repeated eagerness of investors to extrapolate returns and ignore the Iron Law of Valuation has been the source of the deepest losses in history.
A corollary to the Iron Law of Valuation is that one can only reliably use a price/X multiple to value stocks if X is a sufficient statistic for the very long-term stream of cash flows that stocks are likely to deliver into the hands of investors for decades to come. Not just next year, not just 10 years from now, but as long as the security is likely to exist. Now, X doesnt have to be equal to those long-term cash flowsonly proportional to them over time (every constant-growth rate valuation model relies on that quality). A good way to test a valuation measure is to check whether variations in the price/X multiple are closely related to actual subsequent returns in the security over a horizon of 7-10 years.
This is very easy to do for bonds, especially those that are default-free. Given the stream of cash flows that the bond will deliver over time, the future return can be calculated by observing the current price (the only variation from actual returns being the interest rate on reinvested coupon payments). Conversely, the current price can be explicitly calculated for every given yield-to-maturity. Because the stream of payments is fixed, par value (or any other arbitrary constant for that matter) is a sufficient statistic for that stream of cash flows. One can closely approximate future returns knowing nothing more than the following valuation ratio: price/100. The chart below illustrates this point.
[Geeks Note: the estimate above technically uses logarithms (as doubling the bond price and a halving it are symmetrical events). Doing so allows other relevant features of the bond such as the maturity and the coupon rate to be largely captured as a linear relationship between log(price/100) and yield-to-maturity].
Put simply, every security is a claim on some future expected stream of cash flows. For any given set of expected future cash flows, a higher price implies a lower future investment return, and vice versa. Given the price, one can estimate the expected future return that is consistent with that price. Given an expected future return, one can calculate the price that is consistent with that return. A valuation multiple like Price/X can be used as a shorthand for more careful and tedious valuation work, but only if X is a sufficient statistic for the long-term stream of future cash flows.
Margins and Multiples
representative measures of future cash flows when investors consider questions about valuation. Its striking how eager Wall Street analysts becomeparticularly in already elevated marketsto use current earnings as a sufficient statistic for long-term cash flows. They fall all over themselves to ignore the level of profit margins (which have always reverted in a cyclical fashion over the course of every economic cycle, including the two cycles in the past decade). They fall all over themselves to focus on price/earnings multiples alone, without considering whether those earnings are representative. Yet they seem completely surprised when the market cycle is completed by a bear market that wipes out more than half of the preceding bull market gain (which is the standard, run-of-the-mill outcome).
The latest iteration of this effort is the argument that stock market returns are not closely correlated with profit margins, so concerns about margins can be safely ignored. As it happens, its true that margins arent closely correlated with market returns. But to use this as an argument to ignore profit margins is to demonstrate that one has not thought clearly about the problem of valuation. To see this, suppose that someone tells you that the length of a rectangle is only weakly correlated with the area of a rectangle. A moments thought should prompt you to respond, Of course notyou have to know the height as well. The fact is that length is not a good sufficient statistic, nor is height, but the product of the two is identical to the area in every case.
Similarly, suppose someone tells you that the size of a tire is only weakly correlated with the number of molecules of air inside. A moments thought should make it clear that this statement is correct, but incomplete. Once you know both the size of the tire and the pressure, you know that the amount of air inside is proportional to the product of the two (Boyles Law, and yes, we need to assume constant temperature and an ideal gas).
The same principle holds remarkably well for equities. What matters is both the multiple and the margin.
Wall StreetYou want the truth? You cant handle the truth! The truth is that in the valuation of broad equity market indices, and in the estimation of probable future returns from those indices, revenues are a better sufficient statistic than year-to-year earnings (whether trailing, forward, or cyclically adjusted). Dont misunderstandwhat ultimately drives the value of stocks is the stream of cash that is actually delivered into the hands of investors over time, and that requires earnings. Its just that profit margins are so variable over the economic cycle, and so mean-reverting over time, that year-to-year earnings, however defined, are flawed sufficient statistics of the long-term stream of cash flows that determine the value of the stock market at the index level.
As an example of the interesting combinations that capture this truth, it can be shown that the 10-year total return of the S&P 500 can be reliably estimated by the log-values of two variables: the S&P 500 price/book ratio and the equity turnover ratio (revenue/book value). Why should these unpopular measures be reliable? Simple. Those two variablestogether capture the valuation metric thats actually relevant: price/revenue. If you hate math, just glide over any equation you see in what followsits helpful to show how things are derived, but its not required to understand the key points.
price/revenue = (price/book)/(revenue/book)
Taking logarithms and rearranging a bit:
log(price/revenue) = log(price/book) + log(book/revenue)
If price/revenue is the relevant explanatory variable, we should find that in an unconstrained regression of S&P 500 returns on log(price/book) and log(book/revenue), the two explanatory variables will be assigned nearly the same regression coefficients, indicating that they can be joined without a loss of information. That, in fact, is exactly what we observe.
Similarly, when we look at trailing 12-month (TTM) earnings, the TTM profit margin and P/E ratio of the S&P 500 are all over the map. When profit margins contract, P/E ratios often soar. When profit margins widen, P/E ratios are suppressed. All of this introduces a terrible amount of useless noise in these indicators.
As a result, TTM margins and P/E ratios are notoriously unreliable individually in explaining subsequent market returns. But use them together, and the estimated S&P 500 return has a 90% correlation with actual 10-year returns. Moreover, the two variablesagaincome in with nearly identical regression coefficients.
Why? Because they can be joined without a loss of information; that is, the individual components contain no additional predictive information on their own. Just like the area of a rectangle and Boyles Law:
price/revenue = (earnings/revenue)*(price/earnings)
Again, taking logarithms:
log(price/revenue) = log(profit margin) + log(P/E ratio)
The chart below shows this general result across a variety of fundamentals. In each case, the fitted regression values have a greater than 90% correlation with actual subsequent 10-year S&P 500 total returns. Lets be clear hereIm not a great fan of this sort of regression, strongly preferring models that have structure and explicit calculations (see, for example, the models presented in It Is Informed Optimism to Wait for the Rain ).
The point is that one cant cry that profit margins arent correlated with subsequent returns without thinking about the nature of the problem being addressed. The question is whether P/E multiples, or the Shiller cyclically adjusted P/E, or the forward operating P/E, or price/book value, or market capitalization/corporate earnings, or a host of other possibilities can be used as sufficient statistics for stock market valuation. The answer is no.
What we find is that both margins and multiples matter. and they matter with nearly the same regression coefficientsall of which imply that revenue is a better sufficient statistic of the long-term stream of future index-level cash flows than a host of widely followed measures. Emphatically, one should not use unadjusted valuation multiples without examining the relationship between the underlying fundamental and revenues. That is why we care so much about record profit margins here.
Note that in each of these regressions, the coefficients could place a low weight on profit margins and other measures that are connected with revenues, if doing so would improve the fit. They could place significantly different coefficients on margins and multiples, if doing so would improve the fit. They just dont, and like the area of a rectangle and Boyles Law, this tells you that it is the product of the two measures that drives the relationship with subsequent market returns.
[Geeks Note: Gross value added (essentially revenue of US corporations including domestic and foreign operations) is estimated as domestic financial and nonfinancial gross value added, plus foreign gross value added of US corporations inferred by imputing a 10% profit margin to the difference between total US corporate profits after tax and purely domestic profits. Varying the assumed foreign profit margin has very little impact on the overall results, but this exercise addresses the primary distinction (h/t Jesse Livermore) between normalizing CPATAX by GDP versus normalizing by estimated corporate revenues.]
To illustrate these relationships visually, the 3-D scatterplot below shows the TTM profit margin of the S&P 500 along one bottom axis, the TTM price/earnings ratio on the other bottom axis, and the actual subsequent 10-year annual total return of the S&P 500 on the vertical axis. This tornado of points is not distributed all over the map. Instead, youll notice that the worst market returns are associated with points having two simultaneous features: not only above-average profit margins, but elevated price/earnings multiples as well.
This combination is wicked, because it means that investors are paying a premium price per dollar of earnings, where the earnings themselves are cyclically-elevated and unrepresentative of long-term cash flows. This is the situation we observe at present. It bears repeating that the S&P 500 price/revenue multiple, the ratio of market capitalization to GDP, and margin-adjusted forward P/E and cyclically adjusted P/E ratios remain more than double their pre-bubble historical norms.
[Geeks Note: On a 3-D chart where the Z variable is determined by the sum or product of X and Y, a quick way to visually identify the relationship is to view the scatter from either ,max(Y)> or as above].
The upshot is that regardless of the metrics used, S&P 500 nominal total returns in the coming decade are likely to be in the very low single digitsfrom current levels. But remember the Iron Law of Valuationfor a given stream of long-term expected cash flows, as valuations retreat, prospective returns increase. This should be a cause for optimism about future investment opportunities. Unfortunately, not present ones.














