Estimating Timber Depreciation in the Brazilian Amazon
Post on: 7 Июль, 2015 No Comment
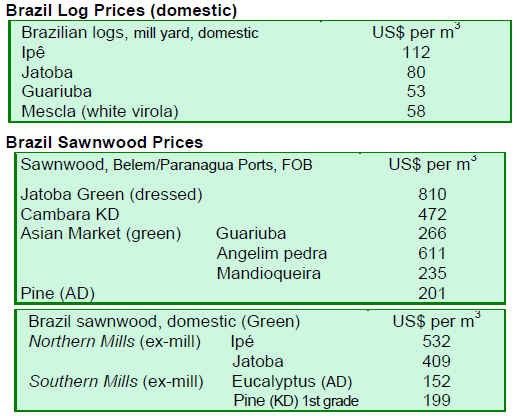
Adjustment in National Accounts
Literature on depreciation accounting procedures of natural resource exhaustion is still open to debate about the most appropriate method. 7 Economic progress has been measured in almost every nation world-wide by the system of national accounts using the Gross National Product (GNP). It uses market prices to measure the aggregate value of an economy’s output in a given year. Despite being the most used indicator of economic progress, it is widely known that GNP is a very poor approximation of economic well being. Another indicator, Net National Product (NNP), is a much better measure of well being because it accounts for the depreciation of the wealth of a country. Man-made capital for example, depreciates through time and this loss in value is captured in the NNP measure. Nonetheless NNP, in its traditional measure, only takes into account the losses of man made capital, leaving aside the depreciation of natural capital. 8 This depreciation happens when the stock of a natural resource is decreased.
The idea of adjusting the system of national accounts to incorporate the losses due to the use of natural resources, is closely related to the concept of sustainability as an indicator of sustainable development. 9 Although there are many definitions of sustainable development, economic definitions have focused on sustainable development as non-declining per capita welfare over time [Pearce and Atkinson, (1995)]. The idea behind it is that every future generation must have the option of being at least as well off as its predecessor. Hence a sustainable path has the characteristic that along it, the overall productive capacity of an economy is not reduced. 10
The productive capacity of an economy depends on the total stock of capital available, as well as on its productivity. If some capital is used up and not replaced, the possibilities of future production is decreased. This is not so much of an issue with physical capital, since it can be produced and replaced. Nevertheless, it is an issue with natural capital which cannot be created by mankind. Therefore, when natural resources are used up or destroyed, there is implicitly a question of substitution.
The question to ask is, at each point in time, how much of the natural capital productive base can be used up? The answer given by economic theory is based on two different approaches developed in a parallel way and yielding theoretically equivalent results. Both aim at measuring the impacts of resource depletion on long run human welfare, but while they are equivalent in theory, they will not yield necessarily the same result in practice. 11
The first approach was derived in Hartwick (1977). He showed that so long as the capital stock of an economy did not decline over time, non-declining consumption was possible. We can illustrate Hartwick’s idea with a simple economy consisting of two types of capital: physical and natural capital denoted by Kt and Rt . respectively. The net investment in this economy is given by the gross investment minus the depreciation in each of the form of capital: . If the net investment is greater than or equal to zero, the country can at least sustain its actual consumption level. If this country produces a non-renewable resource, non-declining consumption is possible by reinvesting all Hotelling rents from the exhaustible resource in physical capital.
These rents are those resulting from an intertemporal efficient extraction programme. This is what is known in the literature as the Hartwick rule. It tell us the amount that we have to invest in other forms of capital when we extract a natural resource and earn rents on it. It was shown by Solow (1986) that it also represents a requirement to keep capital intact. There are two important assumptions implicit in Hartwick’s result. First, physical capital and natural capital are assumed to be close substitutes. 12 Second, the model assumes that an individual only derives utility from the consumption of goods and not directly from the environment.
The second approach was developed from the work of Weitzman (1976) on Net Domestic Product (NDP). We can define NDP for an economy with balanced trade 13 as, . Weitzman (1976) demonstrated that under optimal growth, NDP should be though of as income in the Hicksian sense 14 interpreted as a long run measure of economic well-being, that is, the stationary equivalent of future consumption. In this sense, national wealth for a country would be given by the present value of the best consumption path into the future that society can afford. 15 Weitzman proved that Net National Product (NNP) in any year t is equal to the discount rate (social rate of time preference) 16 multiplied by wealth: NNP(t)= W(t). Based on this result, he argued that a true measure of NDP should include the value of changes in resource stocks. Consequently, the net investment should be defined taking into account the depreciation in all types of capital, not only physical. Weitzman (1976) result depends on two crucial assumptions: first that a country growth path is optimal and second that social welfare equals consumption.
In both of the approaches presented above, we need an estimate of the depreciation of natural capital in order to subtract it from the value of the capital stock. In this manner we would adjust the gross investment to net investment. For an exhaustible resource, Hartwick and Hageman (1993) define depreciation as the change in value of an exhaustible resource under optimal use.
If optimality conditions do not hold and substitution possibilities between physical capital and natural capital are restricted, then, the above approaches face criticisms on the basis of sustainability views. These approaches are regarded as based on the weak sustainability principle which sustains that natural capital can be permanently substituted by physical capital. Consequently, when natural resources are extracted, we can set aside part of its revenue to invest in physical capital and maintain intact the economy’s productive capacity.
This idea is not widely accepted. For some natural resources serving as production factors, there could be thresholds, carrying or assimilative capacity, causing some types of natural capital to be hardly substitutable. Many assets are essential to human being survival in the long run and this point of view is expressed in the strong sustainability concept, based on the conservation of many types of natural capital (i.e. maintaining them intact for future generations) or recognition of their safe minimum standards.
Although depreciation methods can be analysed along these sustainability principles, 17 the proposed depreciation methodologies can be generalized as elaborated in V&H (1997) and summarized in the following sub-sections.
The Change in Value Method
Depreciation of an asset over a period of time can be calculated as the value of the asset in the beginning of the period minus the value of the same asset in the end of the period. This method is known as the change in value method.
Adopting the definition of income as the level of consumption that could be enjoyed without diminishing the capital stock, we can define depreciation as the consumption of the assets in excess of this amount. More formally, depreciation exists when there is degradation of a resource. If we have the value of a resource at the end of a period and the value at the beginning of the period, we can calculate the depreciation of the resource as the difference between the two values. 18 Defining the value of a resource as the discounted sum of the resource rents that are generated over time 19 and using some algebraic manipulation, we can express the depreciation of an asset as: 20
(1)
The previous expression is known as the fundamental equation of asset equilibrium. Although in theory it is a good representation for the depreciation of an asset, carrying out the previous calculation in practice has many complications. We would need projection of the future flow of rents, in which case we need prices, quantities extracted and cost schedules into a future finite stream.
Total Hotelling Rent as Depreciation
Hotelling rent (HR) is defined as the rent that exists on the marginal quantity of an exhaustible resource (price minus marginal cost) and it is considered a measure of the intertemporal scarcity of that resource. This rent exists because the resource is exhaustible and consequently, the owners of the resource extract less than the amount that would equate marginal revenue to marginal cost. Multiplying the HR by the quantity extracted of the resource, gives the total Hotelling rent. It is interpreted as the portion of profit that accrue to extractive firms because they are mining an exhaustible resource.
Hartwick (1988) proved that along dynamically efficient paths of extraction, Hotelling rent and economic depreciation (with a negative sign) are equals. Using this result, it is possible to use a short cut to calculate economic depreciation. Nevertheless, it is important to note that this equivalence is only correct under certain conditions, namely: a ) resource extracted optimally, i.e. Hotelling’s rule holds; b ) price of extracted resource constant over time; c ) marginal cost as an increasing function of the amount extracted, unrelated to the size of the reserve and constant over time; and d ) constant discount rate over time.
The Net Price Method (NPM)
As it was mentioned previously, under special conditions, economic depreciation of natural resources can be approximated by the Hotelling rent. This last term was defined as price minus marginal extraction cost (the profit on the marginal ton extracted), multiplied by the amount extracted. Hence we can use the following expression to calculate the depreciation of natural capital from the NPM:
(2)
In theory this method would be easier to apply than the change on value method. Nevertheless in practice, the data on marginal extraction cost is not generally available. Consequently previous studies that attempted to calculate depreciation of natural capital used the so called net price method. It uses data on average cost instead of marginal cost and calculates the depreciation as:
(3)
It is important to note that, as it was mentioned by several authors, 21 using the average net price instead of the marginal net price, one is calculating the total resource rent which overstates net accumulation (unless the MC=AC as in the case of a linear cost function).
The El Serafy Method (ESM)
Alternatively to the net price method, El Serafy (1989) developed a method to calculate depreciation based on the concept of user cost. He equates the finite stream of rents earned by a resource to an annuity X earned forever, which can be obtained by selling the mine and depositing the value of the mine (V ) in a bank account. Mathematically this is given by:
(4)
where r is the discount rate, n+t is the last year of the extraction, X is the annuity received and R is the rent defined as . The expression R-X is a measure of depreciation, and El Serafy (1989) simplifies further the analysis to obtain a closed form solution.
Assuming that the rent is constant over time, 22 such that , the equation above is simplified to:
(5)
The previous expression is the user cost or economic depreciation for the ESM. It is important to note that as Hartwick and Hageman (1993) pointed out, if R(t) changes over time, the measure R-X could be a poor approximation to R(t+i)-X in period i. consequently the ESM would yield a poor approximation of the depreciation value.
In the ESM, n is the remaining years of life of the resource stock. This is estimated by dividing total reserves remaining by the year’s quantity extracted. This calculation implicitly assumes that this year’s extraction will continue into the future at the same rate. If S(t) is the remaining stock of the resource at period t and q(t) the quantity extracted of the resource in the same period, we can express ESM formula to estimate depreciation as:
(6)
Vincent and Hartwick Approach
Vincent and Hartwick (1997) suggested a transformation applied to the average cost in order to get a consistent estimate of depreciation. If the marginal cost curve is isoelastic in the quantity extracted and assuming a functional form for the total extraction cost as they show that MC=( 1+ )AC. Therefore we can express the expression for the Hotelling as:
(7)
Where is the elasticity of the marginal cost curve with respect to the quantity extracted. It is important to note that on the previous formula, for relative elastic marginal cost curves (1), unit price has to be at least twice as large as the unit average cost. Otherwise, one would witness a negative estimate of depreciation which would not make economic sense in presence of increasing depletion. This arithmetical trap is only due to the simplification of the isoelastic assumption of the marginal cost curve.
Another approach used by Vincent (1997), is to use the previous total cost function and the fact that under an optimal extraction path, the transversality condition implies that MC=AC when the resource is exhausted. As a result, it is shown that for the previous functional form, this transversality condition occurs if and only if q(t)=MC=AC= 0 in the final period.
Using the Hotelling rule and the fact that MC(T)= 0, Vincent (1997) derives the following expression for the Hotelling rent:
(8)
The previous expression is a generalization of the net price and El Serafy methods in the sense that it embodies both estimations as particular cases. If , equation (8) simplifies to the ESM and If , the whole right hand side goes away and the Hotelling rent would just equal the total rent.
The usefulness of the previous formula is that in practice it is much easier to find data available for the average cost than for the marginal cost. However, estimating can be a practical complication. Obviously the elasticity of marginal cost will vary depending on the nature of the resource (timber, oil, etc.) and type of industries. 23
Equations (7) and (8) are presented by Hartwick and Vincent (1997) as being equivalent. The choice of which one to use depends just on the availability of data. If data is obtainable on the average extraction cost, then one can use expression (7) to estimate the depreciation. If instead, data is available on the resource rent, Vincent (1997) has demonstrated that expression (8) is an alternative measure of the Hotelling rent. Nevertheless it is noted in Vincent (1997) that expression (7) would yield less accurate estimates of economic depreciation than formula (8). This would happen due to the fact that formula (7) is based on current marginal rent and, on the other hand, expression (8) is based on long run principles.
Although Vincent (1997) is correct in his concern for estimating long run instead of short run Hotelling rents, it is not clear in his argument why the formula from expression (8) would take into account out of equilibrium behaviour in the timber market. Since it also uses current price and costs of logged wood, any tendency of resource prices to fluctuate in the short run will be incorporated into his estimates using expression (8) as well as expression (7).
Moreover, since expression (8) incorporates a discount rate and formula (7) does not, any bias caused by fluctuation in prices could be compensated by biases caused by the choice of an incorrect discount rate. Consequently, it does not seem to exist any a priori reason why biases in the discount rate chosen would not be even worse than biases from price fluctuations or out of the equilibrium behaviour in timber markets.
In spite of previous points, our estimates of depreciation values will follow equation (8) and we will be assuming additionally permanent conversion of forest land to agriculture purposes. This would imply that we will not be considering any form of reforestation or second growth forest conversion.














