Convertible bond hedging typically involves purchasing a convertible security and shorting the stock into which it is convertible

Convertible Bond Hedging

Convertible bond hedging typically involves purchasing a convertible security and shorting the stock into which it is convertible. Shorting reduces the investors exposure to changes in the stock price, because price movements in the convertible are at least partially offset by the price movements of the short stock position. More sophisticated variants include hedging so that the net expected position is fully hedged with respect to changes in the stock price, or hedging so that the net expected position is also fully hedged with respect to changes in interest rates and/or credit spreads.

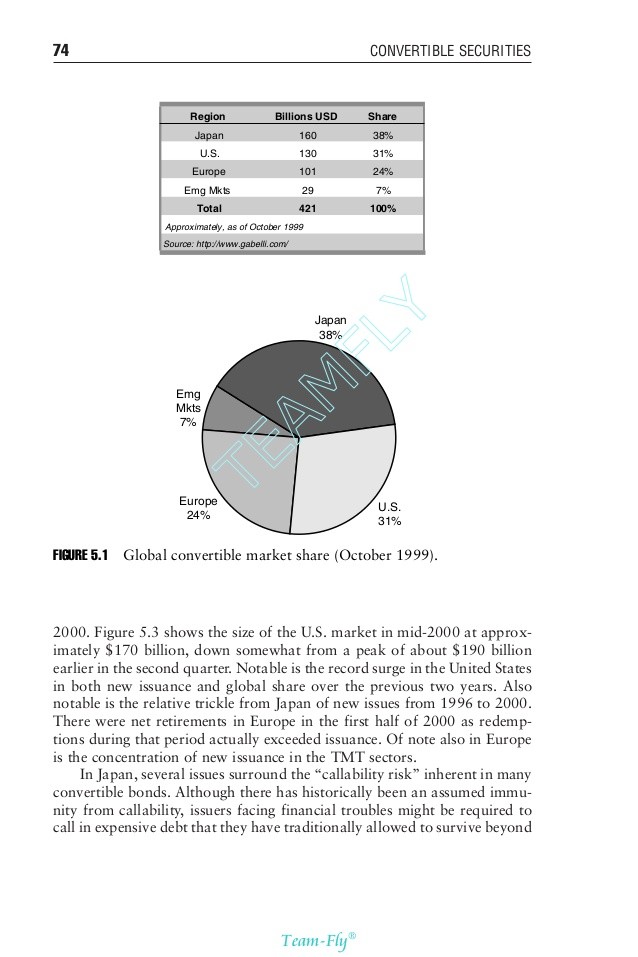
Convertible hedging has been around for years. Warren Buffett is reported as saying: I got my start at age 21 arbitraging convertible bonds against the underlying securities.1 The reported returns generated

by the strategy are relatively stable, averaging 13% to 16% per year on a leveraged basis, with relatively few periods of negative performance.2

This chapter reviews the basic strategy, provides results from a study of convertible bond hedging, and raises several practical implementation issues.

CONVERTIBLE SECURITIES

There are two basic types of convertible securitiesconvertible bonds and convertible preferred stock. A convertible bond is a bond issued by a corporation that can be converted (typically) into shares of the stock of that corporation. The investor converts the bond by surrendering it to the corporation or its agent and receiving shares in the company.

A convertible bond, like other bonds, has a maturity, a coupon rate, and a call schedule. In addition, because it is convertible, it has a conversion ratio, which gives the number of shares into which it is convertible. For example, if the conversion ratio is 30, the bond can be converted into 30 shares of stock. The conversion ratio can also include a fractional amount, indicating that the bond is convertible into less than one full share of stock.

Although a convertible bond is usually convertible into shares of the issuing company, this is not always the case. Company X, for example, may own a significant amount of stock of Company Y. It may decide to liquidate its holdings of Y by issuing convertible bonds that are convertible into shares of Company Y. Bonds that are convertible into shares of a company other than their issuer are commonly referred to as exchangeable securities. There are also convertible bonds that are redeemable for other bonds, such as U.K. government gilts.

Exhibit 4.1 graphs a hypothetical convertible bond. The maturity on the bond is seven years, and it pays an annual coupon of 10%. It is convertible into 20 shares of stock of the issuing company. The current interest rate on the issuers bonds is 10%.

The graph shows three lines. The dotted line is the value of the bond-only part of the security. This is often referred to as its bond value. Bond value is usually stable, reflecting the maximum amount the bondholder can earnthe interest on and the face value of the bond. Even if the value of the company increases, as evidenced by rising share price, the bond value stays level. Note, however, that if the companys value falls sufficiently, so that the company nears bankruptcy, the bond value declines. This reflects the fact that, in the event of bankruptcy, bondholders may not fully recover the face value of their bonds.

The dark solid line in Exhibit 4.1 is the securitys conversion value the value of the security if it is converted into stock. This line is obtained by multiplying the conversion ratio by the share price. The conversion value thus rises and falls with the value of the companys stock.

Because the holder of the convertible can either ignore the conversion option and hold the bond until maturity or convert it, the convertible has to be worth at least the higher of its bond-only value or its conversion value. In fact, the convertible is actually worth more, because the convertible holder has the option to convert the bond at his or her discretion. The light solid line in Exhibit 4.1 gives the value of the convertible reflecting this option.

Why is the convertibles value greater than either its bond value or its conversion value? First, assume the companys share price is low, so that the convertible holder would not choose to redeem the convertible for stock, but would rather keep the bond and receive its face value at maturity. (More formally, the convertibles current bond value exceeds its conversion value.) There is the chance, however, that the stock price could rise substantially at some point prior to the maturity of the bond, so that the bondholder would want to redeem the convertible for the stock. (More formally, the bonds conversion value exceeds its bond value.) As long as there exists some chance of converting favorably into stock, the convertible must trade for more than the otherwise identical straight bond represented by its bond-only value.

Second, assume the stock price is high and the convertibles conversion value exceeds its bond value. It would seem to make more sense to convert the bond into stock rather than hold it to maturity and redeem it for face value. In this case, however, there is the chance that the stock price could fall substantially before the convertible reaches maturity; if that were to happen, the convertible holders downside would be limited by the convertibles bond value. An investor would therefore prefer the convertible to an unprotected stock position equal in value to the convertibles conversion value.

Clearly, the additional amount a buyer of a convertible is willing to pay over either its bond-only value or its conversion value depends upon the likelihood that the conversion option will be exercised. This likelihood, hence the value of the embedded conversion option, will be greatest at the intersection of the bond-only value and the conversion value.

DELTA AND DURATION

Two concepts facilitate discussion of convertible securitiesthe likely change in the value of the convertible given a change in the price of the stock, and the likely change in the value of the convertible given a change in interest rates. The first concept is often referred to as delta. More rigorously, the delta of a convertible is defined as the convertibles rate of change with respect to a change in the stock price. Mathematically, it can be written as:

C is the value of the convertible and S is the price of the stock.

As the underlying stock price rises, the bonds conversion value increases. As the convertibles value approaches its conversion value, the bond is said to become deeply in-the-money. As this happens, the convertibles delta approaches one, meaning that the convertible begins to move one-to-one with the stock price. As the stock price falls, the convertible moves out-of-the-money. The convertibles delta approaches zero and the convertible behaves more and more like a bond, with small changes in stock price having little effect on its value.

The second conceptthe likely change in value of the convertible, given a change in interest ratesis captured by the bonds duration.3 Mathematically, duration can be defined as:

= ( ?C ?r? ) C?

C is the value of the convertible and r is the interest rate.

Exhibit 4.2 illustrates the value of the convertible and the bonds duration if interest rates change by 100 basis points. The dashed line represents the value of the convertible when the general level of interest rates is 10%. The solid line represents the value of the convertible when the general level of interest rates is 9%. It is obvious that interest rate

changes will have a greater effect on the convertibles price when the convertible is out-of-the-money than when it is deeply in-the-money.

It should be noted that these relationships break down when the issuing companys share price declines drastically. In that event, there is significant credit riskin other words, the potential that the company may go bankrupt. The convertible then becomes what is known as a busted security. Its pricing is driven by liquidation, or recovery, values, rather than either the companys stock price or interest rates. Busted convertibles are traditionally treated as part of the distressed security universe, rather than as hedgeable convertible bonds.

In summary, when the convertible is deeply in-the-money, it is very sensitive to changes in stock price and not very sensitive to changes in interest rates. When it is out-of-the-money, the reverse is true (barring fears of bankruptcy). These two concepts of delta and duration drive convertible bond hedging.

HEDGING CONVERTIBLES

Convertible bond hedging typically involves purchasing a convertible bond and shorting an appropriate amount of the issuing companys stock so that the net position is delta neutral. Being delta neutral means that if the underlying stock price were to move, any change in the value of the convertible would be offset dollar for dollar by a change in the value of the short stock position. More sophisticated variants of convertible bond hedging include hedging the convertibles interest rate risk by shorting interest rate futures so that the combined position is duration neutral as well as delta neutral.4

The March 1, 1993 issue of Value Line Convertibles offers an example. The Staples Inc. 5% coupon convertible bond due in 1999 is purchased at a price of $965. The appropriate delta-neutral stock hedge ratio, according to Value Line, is 0.40. That is, for every dollar invested in the convertible, 40 cents of the underlying stock should be shorted. With Staples stock trading at $31.50 per share, one would short 12.3 shares of stock for each convertible purchased. Value Line Convertibles gives the appropriate interest rate hedge ratio as $2.09 for a 100-basispoint shift in interest rates. This would require shorting 0.00209 of a five-year futures contract.

Exhibit 4.3 shows the computation of the return on a portfolio comprised of a long position in the Staples convertible bond plus a short position in the underlying stock. This computation assumes no movement in the underlying stock price, hence is often called the standstill or static rate of return. The standstill rate can be thought of as the cost-ofcarry of the trade. In this case, it is the coupon income on the bond plus the short rebate on the proceeds of the short sale minus the dividend yield on the shares sold short. For the Staples position, the annualized standstill rate of return is 6.19%.

What if the Staples stock were to move, while interest rates remained unchanged? Exhibit 4.4 gives the cash flows in this case. Whether the stock moves up by $1.00 or down by $1.00, the overall portfolio value remains essentially unchanged.5 The portfolio, which had a value of $579 when the

underlying stock was priced at $31.50, is worth $579.25 if the stocks price falls by one dollar and $579.75 if the stocks price rises by one dollar. The short stock position hedges the portfolio against changes in the convertible bonds value resulting from changes in the underlying stock price. A short position in interest rate futures would similarly hedge the portfolio against changes in the convertible bonds value due to changes in interest rates.

The Staples example omits items such as transaction costs and focuses on only one security at one point in time. In a broader context, and accounting for transaction costs and other factors, a convertible hedging strategy should yield no excess returns if markets are efficient.

If markets are efficient, all assets are fairly priced and there are no arbitrage opportunities offering abnormal returns.

AN EMPIRICAL ANALYSIS

Can convertible bond hedging yield excess returns? I constructed a portfolio of convertible bond trades for the period January 1989 to July 1996. This time period was chosen because it included both up and down markets in stocks and bonds.

Data were collected for each of the 90 months using Value Line Convertibles as the primary source. In order to simplify the process, and to better reflect real-world trading conditions, only convertible issues of at least $100 million in size were included in the sample.

The portfolio started out with equally weighted positions in all available convertible bonds and preferred stocks over $100 million in issue size. The portfolio was rebalanced monthly. On average, it included 146 convertible securities.

To hedge the portfolios stock price exposure, each convertibles underlying stock was shorted in the amount given by Value Line. To hedge the portfolios interest rate exposure, five-year Treasury note futures were shorted in the amount specified by Value Line.

The portfolio accrued coupon income over the course of each month. Dividends owed as a result of the short sale of stock were also accrued in order to facilitate computations. The portfolio was assumed to earn a short rebate equal to 85% of the three-month Treasury bill return on the dollar amount of the proceeds from the short sales.

Transaction costs were assumed to be $0.10 per share on both the sale and purchase of stock. Convertible bond and preferred transaction costs were assumed to be one full point ($10 on a standard $1,000 face bond) on both purchases and sales. The transaction cost for a five-year Treasury future was assumed to be $20 per contract on a round-trip basis.

Results

For the period, the average monthly return on the portfolio was 75.53 basis points, or 9.06% per year (on an unleveraged basis). The average monthly excess return over Treasury bills was 30.37 basis points, or 364 basis points per year. In only 19 of the 90 months were the total returns negative.

On the surface, these results appear to suggest that there are inefficiencies in asset pricing that can be exploited by convertible bond hedging. In fact, the data suggest that the inefficiencies are so large that it is possible not only to generate significant alpha, but to do so with a high degree of consistency. One must ask whether an incorrect assumption in the analysis, or hidden risk, can explain this.

Perhaps the analysis underestimated the impact of transaction costs. To test this possibility, I repeated the analysis using various levels of transaction costs. More precisely, I asked how large transaction costs would have to be in order to eliminate all the alpha. Bringing returns down to Treasury rates of return required abnormally large assumptions for transaction costs, on the order of $0.69 per share for a stock purchase or sale. It seems unlikely that underestimation of transaction costs can account for the excess returns to the hedged convertible bond portfolio.

Do the excess returns represent a compensation for bearing risk? Perhaps the portfolio was not perfectly hedged to be delta neutral and/or duration neutral. If the portfolio were not hedged correctly, the excess returns would represent compensation for residual interest rate or stock market risk. To test this possibility, I regressed the hedged convertible bond returns on both stock and bond indexes. The results indicated that the hedged portfolio had no net exposure to either the stock or the bond market.

In summary, it would appear that, over this period at least, investors could have attained significant excess returns by investing in and hedging convertible securities. In fact, this period saw the operation of several hedge funds dedicated to the strategy.

IMPLEMENTATION ISSUES

When implementing a hedging strategy, several important issues arise. First, how does one determine the composition of the hedge? Second, how important is credit risk, given that convertibles are typically very junior securities in the capital structure? Finally, what about practical issues such as the availability of stock to borrow in order to execute necessary short sales?

Determining the composition of the hedging portfolio requires two steps: (1) using a model to determine the amount of securities or derivatives that should be shorted and (2) using judgment to modify this amount, when appropriate. There are many software packages that can evaluate convertibles and give the appropriate hedges. However, behind these programs are models dependent on several difficult to estimate variables, including the future volatility of underlying stock prices and the likelihood of an issuer calling a given convertible. Investors must make judgments about these variables. Different individuals using the same software can thus come up with very different hedging portfolios.

Furthermore, an investor may prefer a less than full hedge of the convertible portfolios delta and/or duration risk. For example, if the investor believes that interest rates are going to decline, he or she may want to retain some exposure to interest rate risk so that the portfolio can profit if the expectation of falling rates turns out to be correct. Many convertible hedgers retain some delta exposure so that they can profit from the long-term upward trend of stock prices.

Another issue involves busted securities. When an issuer faces credit trouble, its convertibles may be the first to feel it, as they are usually the most junior debt security. After paying off more senior debt, the issuer may not find much left on the left-hand side of the balance sheet to cover the value of its convertibles. As a result, convertible prices can fall dramatically when the specter of bankruptcy raises its head.

An abrupt decline in a convertibles price due to fears of bankruptcy creates particular problems for the convertible hedger. As we noted earlier, the convertibles delta, which represents the amount of stock to be sold short against the convertible, normally approaches zero as the convertible moves further and further out-of-the-money (that is, as its conversion value declines). When there is a threat of bankruptcy, however, delta increases toward one and in fact may at times exceed one. This is because the convertibles bond value starts to approach zero, leaving only its conversion value (the stock price).

As bankruptcy fears begin to materialize, the convertible hedger may have to sell substantial amounts of stock short. Of course, other investors will also be selling the stock, or selling it short, driving its price down. Given the uptick rule (i.e. no short sales on a downtick), shorting may become impossible or at least impractical. The investor should thus do some credit research in order to avoid purchasing potential busted securities in the first place.

Several other practical problems arise in relation to short selling. For example, it may be difficult to borrow some securities in order to sell them short. Even if the stock can be borrowed, the short seller faces the risk that the stock may be subject to a buy-in. If the broker cannot find other shares to substitute for the ones called in, the convertible position may be left unhedged or only partially hedged.

Leverage presents another set of problems. Regulation T, covering equity investments, allows an investor to purchase $1.00 worth of stock long and to sell short $1.00 worth of stock for every $1.00 of equity capital. But the margin rules on hedged convertibles differ from the standard stock margin rules. A long convertible position combined with its corresponding short position is effectively treated as zero net investment, because the convertible holder can convert the bond into the shares sold short. Convertible bond hedgers may thus be able to leverage up by twice as much as equity investors. Furthermore, if one is operating outside the purview of Regulation Tas a broker-dealer or hedge fund, sayeven higher leverage is available. In fact, some hedge funds have leverage levels corresponding to a long convertible value of up to 13 times the equity capital in the account.

Leverage will magnify gains from convertible hedging, but it will also magnify losses. In addition, brokerage firms may increase margin requirements at higher levels of leverage. The investor may thus be subject to financing costs, as well as incurring the normal costs associated with financing a highly leveraged position.

A FINAL NOTE

Both anecdotal evidence and more rigorous studies suggest that convertible hedging can generate returns in excess of the risk-free rate, and has done so for decades. In fact, the returns of many convertible bond hedge funds suggest that this phenomenon has continued in recent years. These excess returns do not seem to be explainable in terms of transaction costs or in terms of imperfect hedging. They may nevertheless represent a compensation for bearing less discernible sources of risk.

One hypothesis that has been suggested is that the excess returns represent compensation for bearing liquidity risk. In this view, convertible hedgers are price-takers rather than price-makers. They respond to other investors demands to sell or buy positions. These investors pay up to execute, and the excess returns to convertible hedgers really represent a premium for providing liquidity. The returns to a convertible hedging strategy may thus depend upon the degree of price-taking in markets, and on the hedgers willingness to bear liquidity risk. This hypothesis would seem to be supported by the performance of convertible bond hedge funds during the liquidity crises in 1987, 1990, 1994, 1998 and, most recently, 2002. These funds generally experienced negative quarters corresponding to the market turmoil.

Nevertheless, the evidence from the past several decades indicates that a strategy of purchasing convertible securities and hedging their stock and interest rate risks has been profitable. Investors willing and able to deal with the complexity of convertible bond hedging should consider the strategy as a source of potential alpha.

NOTES

1 Forbes, November 23, 1992.

2 For example, see Pacific Alternative Asset Management Companys database as well as other publicly available databases on convertible hedge funds. 3 Alternatively, duration is sometimes referred to as rho.

4 Convertibles with significant interest rate risk and little stock risk are rarely candidates for hedging.

5 The reason that the return is not exactly zero is that an embedded option is being hedged through time, and the closer the convertible gets to maturity, the less valuable the conversion option becomes. This is known as time decay. The slight positive return generated offsets the effect of time decay.

6 These returns are hypothetical results based on a simulated backtest. Hypothetical

results do not represent actual trading and may not reflect the impact that material economic and market factors might have had on the decision-making process underlying an actual portfolio. Furthermore, the returns, while net of estimated transaction costs, do not reflect management fees; actual client returns would have been reduced by such fees and other expenses.