by Joe Lan, CFA

Valuing a stock or company is one of the most difficult tasks in investing.

Even the most seasoned investors may shy away from the challenge for a variety of reasons. However, determining whether a stock is trading at an attractive valuation is paramount to investment success. In this installment of the Financial Statement Analysis series, I continue to review valuation metrics, specifically the dividend discount model, which is one of the many methods used to determine fair value. I also delve into some of the challenges associated with calculating intrinsic value using this model.

The valuation (stock price) obtained using these formulas can vary substantially, so it is difficult to use the figures as exact buy or sell prices. However, there are several benefits that you can gain by valuing stocks yourself. It forces you, as an investor, to place a specific price on a stock that can be used as a gauge. Perhaps more importantly, valuing stocks enables you to take a deeper look at factors that drive stock price. Characteristics such as growth and fundamental elements such as income play a huge role in stock price value. You will be able to see how these metrics affect the share price determined through the dividend discount model.

Intrinsic Value

Intrinsic value is the underlying or fair value of a company, determined through fundamental analysis. Warren Buffett has become arguably the greatest investor of all time by being able to pick stocks that have a higher intrinsic value than their current market value.

Intrinsic value is frequently calculated by discounting a set of future cash flows or income expected to be generated by a company or stock back to its present value. In other words, present value represents the current value or worth of future cash flows.

For example, $1,000 today is worth more than $1,000 dollars five years from now because of the time value of money. (Inflation erodes the future ability to buy goods and services for the same amount of dollars.) This is why investors would rather receive a sum of money today than wait for an uncertain amount of future cash flow without being adequately compensated for taking the risk.

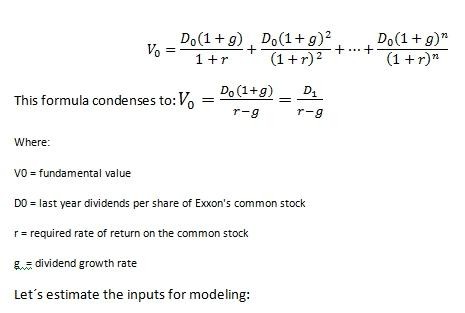
The dividend discount model uses dividends (or income) to generate an intrinsic value. The formula takes the future expected dividend stream of a company and discounts it back to its present value. There are numerous variations of the dividend discount model and we discuss two of the more basic, but more easily calculated by individual investors, in this article. Note that the dividend discount model will not work for companies that do not pay a dividend.

Constant Growth Model

The constant growth dividend discount model assumes that a company is growing at a constant rate. It is best used for large, stable companies that have consistent earnings and dividends. However, small- and medium-sized firms that are growing their earnings and dividends steadily can be valued using this approach as well. The formula for the constant growth model is:

Stock Price = D₁ (k g)

Where:

D₁ = dividend for the coming year>

k = required rate of return; k must be>

g = growth rate of dividends

As with any model, the output generated is only as good as the quality of the factors going into the calculation. Dividends and earnings information is widely available, but the required rate of return and growth rate of dividends require assumptions to be made.

SPECIAL OFFER: Get AAII membership FREE for 30 days!

Get full access to AAII.com, including our market-beating Model Stock Portfolio, currently outperforming the S&P 500 by 4-to-1. Plus 60 stock screens based on the winning strategies of legendary investors like Warren Start your trial now and get immediate access to our market-beating Model Stock Portfolio (beating the S&P 500 4-to-1) plus 60 stock screens based on the strategies of legendary investors like Warren Buffett and Benjamin Graham. PLUS get unbiased investor education with our award-winning AAII Journal. our comprehensive ETF Guide and more – FREE for 30 days

A common formula for estimating the possible required rate of return is:

Required rate of return for equity = risk-free rate + (market risk premium beta for equity)

The risk-free rate used in this calculation is the yield on long-term Treasuries, such as a 30-year Treasury bond. The reasoning behind using a long-term bond is that equities are thought of as indefinite holdings; therefore, the risk-free rate should be a very long-term risk-free rate. According to Ibbotson Associates, long-term Treasuries annualized total return from 1926 to 2012 was 5.7%.

The market risk premium is the expected return of the stock market less the risk-free rate. In short, it is the return required to entice investors to purchase risky assets instead of simply purchasing risk-free assets. A good estimate of this figure is the historical market risk premium of the S&P 500 index. According to Ibbotson Associates, the S&P 500 gained 9.9% annually from 1926 to 2012. Therefore, the market risk premium is 4.2% (9.9% 5.7%). It is worth noting that there are a number of economists who believe our stock market will not be able to achieve the same type of returns we have experienced over the long run. However, this opinion is hard to substantiate, and using a long-term historical market risk premium is considered a sound procedure.

Lets look closely at an example using Microsoft Corp. (MSFT) which closed at $36.56 per share on February 7, 2014. Stock Investor Pro, AAIIs fundamental stock screening and research database, shows a three- to five-year expected growth rate for continuing earnings (based on six analysts) of 7.5%. Earnings from continuing operations are used because this figure excludes one-time expenses and income and the figure is more representative of the companys core business operations going forward. The long-term projected earnings growth rate serves as a proxy for future dividend growth, assuming the payout ratio stays unchanged.

In 2013, Microsoft paid $0.92 per share in dividends. Assuming a 7.5% growth rate, the company will pay $0.99 per share ($0.92 1.075) in 2014. The 7.5% growth in dividends assumes that dividends are growing at the same pace as earnings, which means the payout ratio is staying the same. The figure $0.99 per share is used for D₁ in the dividend discount model.

Beta, the only other figure needed, is a measure of a stocks volatility relative to the market. A beta of 1.0 means the stock is as volatile as the market. Stocks with betas over 1.0 are more volatile than the market, whereas stocks with betas less than 1.0 are less volatile than the market. According to Stock Investor Pro, Microsoft has a beta of 0.97.

Using the above-mentioned risk-free rate and market risk premium, the required rate of return (k) for Microsoft is:

= 5.7% + (4.2% 0.97)

= 9.8% (rounded)

Using a rounded 9.8% required rate of return, the estimated dividend next year and the expected growth rate, the calculated stock price of Microsoft using the constant growth model is:

= $0.99 (0.098 0.075)

This valuation for Microsoft is significantly higher than the current stock price of $36.56, leading to the assumption that Microsoft shares may be undervalued.

It is important to keep in mind that there are shortcomings in the constant growth model. While a growth rate of 7.5% does not seem overly ambitious, the U.S. economy only grew at 3.2% annually from 1947 through 2013, according to Trading Economics. Using 7.5% for the constant growth rate for Microsoft implies that the company will grow at more than double the rate of the U.S. economy in perpetuity, which is likely an unsustainable pace.

In addition, the risk premium used is also an assumption based on the historical market risk premium. Changes to either of these rates will greatly affect the outcome of Microsofts valuation. For example, if the market risk premium is raised to 5.2% and the expected growth rate of Microsoft is lowered to 6.5%, mere 1% changes to both inputs, the valuation drops dramatically. In this example, the rounded required rate of return is:

= 5.7% + (5.2% 0.97) = 10.7%

Assuming a dividend next year of $0.98 based on the 6.5% expected growth rate results in a new calculated price of Microsoft of:

= $0.98 (0.107 0.065)

= $23.33 per share

Multi-Stage Growth Model

An alternative to the constant growth model is the multi-stage growth model. This model is more complicated and requires more estimates. It can, however, account for anticipated future changes in growth rates.

In our previous example, the assumption was that Microsoft would grow at a 7.5% pace into perpetuity. The growth rate used was the three- to five-year expected growth rate found in Stock Investor Pro. Since we know this pace of growth will be difficult to sustain indefinitely, we can update our model by estimating that Microsoft will grow at a 7.5% pace for the next four years and then grow at a rate of 4.5% over the long run, a slightly faster rate than the historical average growth rate of the U.S. economy. I used this figure because, in addition to the U.S. economy, Microsofts business operations are affected by many other faster-growing economies, such as emerging markets.

The calculation for the multi-stage growth model involves adding the present value of dividends paid during the high-growth period to the present value of the companys terminal value.

Table 1. Calculating Present Value of Dividends and Terminal Value