In the days before computers were common, banks used to compound interest quarterly. Four times a year everyone would have their balance increased by one fourth of the nominal interest rate, an exercise that required all bank employees to stay up half the night working this out by hand.

Today, it is possible to get computers to calculate interest monthly, weekly or even continuously as required.

The effect of shorter compounding periods is to increase the return investors get, because the more often rates increase the sooner you get to earn interest on your interest.

There are two measures of interest that can be expressed, and it is important to be able to convert between the two in order to know what your actual return is. The nominal interest rate is quoted as a percentage and a compounding period. The interest per compounding period is the nominal rate divided by the number of periods in a year. For example, a nominal 12% compounded monthly is credited as 1% per month.

You need to allow for the number of compounding periods when comparing two nominal interest rates, for example 12% compounded semi-annually is obviously not going to be as good as 12% compounded daily.

The effective interest rate shows you the actual return from a given interest rate and compounding period, and you can compare one effective interest rate with another without need for any further calculations.

When interest is compounded annually the nominal interest rate equals the effective interest rate.

Using a financial calculator you can readily convert nominal and effective interest rates but if you don’t have a financial calculator the rest of this article gives the formulae for doing so, they aren’t that difficult.

To convert a nominal interest rate to an effective interest rate you apply the formula:

Effective annual rate = (1 + i/m) m — 1

Where i is the nominal annual interest rate and m is the number of compounding periods. For example the effective rate of return of a 15% monthly compounding investment is (1 + .15/12) 12 — 1 = (1 + .0125) 12 — 1 = .1608 = 16.08%

Continuous compounding is possible. This is the limit of compounding where rather than annual, monthly, weekly, daily or even hourly compounding, interest accrues continuously.

Effective annual rate = e i — 1 Where i is the nominal interest rate and e is a mathematical constant with an infinite number of decimal places that equals 2.71828 to five decimal places. If you want to know how I got this formula you’ll need to know calculus, it is the so called limit as m tends to infinity of (1 + i/m) m. If you don’t know or don’t want to know calculus then don’t worry about it, just accept that the effective interest rate is 2.71828 raised to the power of the nominal interest rate.

What is the effective annual interest rate when you have money nominally compounding at 10%pa?

e 0.10 = 0.1052 = 10.52% Putting this into a present value formula:

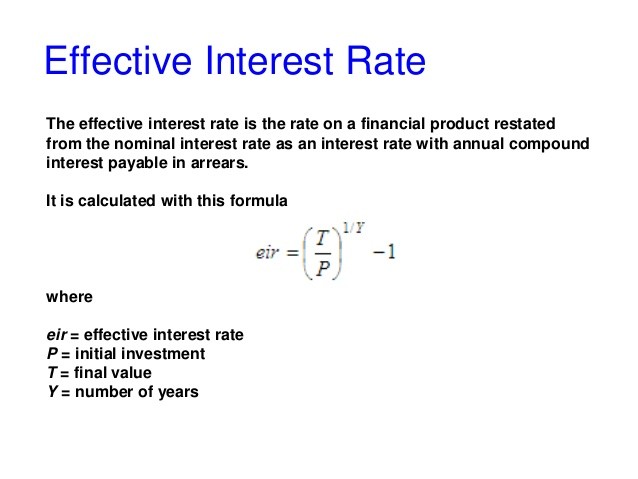
FV = PV e it Where FV = future value (or account balance a time t)

PV = present value (or account balance at the start)

i = nominal interest rate and

t = time in years to invest.

This next bit is optional for someone that doesn’t want to know about calculus, but I have explained things in small enough steps that any high school graduate that did a bit of calculus should be able to follow the thread.

Using calculus it can be proven that this is the correct way to express continuously compounding interest, where FV = the balance at time t and PV is the present account balance.

d/dt means taking the derivative with respect to variable t, which means the same thing in this case as to find the rate at which FV changes over time. Now in basic calculus we know that e has some unusual properties, when you find the derivative with respect to x of e raised to something the derivative always equals the exponent’s derivative with respect to x, multiplied by e raised to the exponent. So d/dx e x = just e x because d/dx x = 1. However d/dx e 2x = 2x x because d/dx 2x = 2.

Basic calculus lesson aside, here is the derivative of this FV formula: