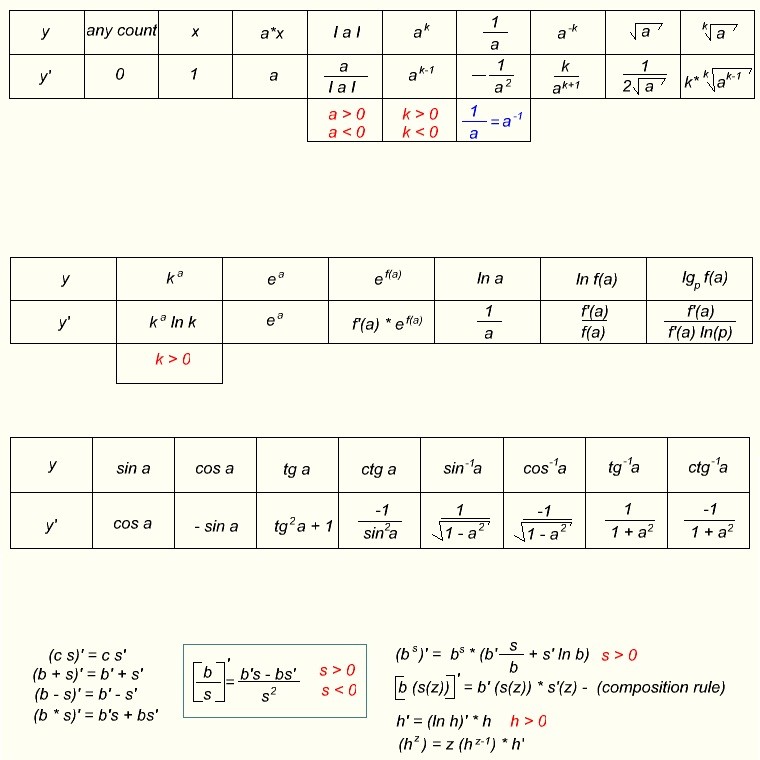
Basic Derivatives
Post on: 25 Июль, 2015 No Comment
Description
INTRODUCTION TO DERIVATIVE SECURITIES ECI RISK TRAINING www.ecirisktraining.com Alan Anderson, Ph.D.
2 DERIVATIVE SECURITIES A derivative security is a contract whose value is based on (derived from) an underlying financial asset Derivatives are widely used as hedging instruments due to their flexibility (c) ECI Risk Training 2009
3 Derivative securities are traded on organized exchanges, such as the Chicago Mercantile Exchange (CME) They are also traded directly between counterparties in the “over-the-counter” (OTC) market (c) ECI Risk Training 2009
4 The basic categories of derivative securities are: forward contracts futures contracts swaps options (c) ECI Risk Training 2009
5 FORWARD CONTRACTS A forward contract is an agreement between two counterparties to exchange an asset at an agreed-upon price at a specified point in the future (c) ECI Risk Training 2009
6 For example, suppose that a U.S. importer needs to buy £100,000 in six months In order to hedge the risk that the dollar will depreciate against the pound in six months, which would increase the dollar price of the pounds, the importer can enter into a forward contract with a bank in which: (c) ECI Risk Training 2009
7 the importer agrees to buy £100,000 from the bank at a fixed price in six months (this is known as a long forward position) the bank agrees to sell £100,000 to the importer at a fixed price in six months (this is known as a short forward position) (c) ECI Risk Training 2009
8 Suppose that the fixed price, known as the delivery price, is $1.60/£. This means that in six months, the importer will pay $160,000 for the £100,000 regardless of the exchange rate that prevails at that time (c) ECI Risk Training 2009
9 In six months, if the dollar has depreciated (i.e. the exchange rate has risen), the importer will save by buying pounds through the forward contract (c) ECI Risk Training 2009
10 In six months, if the dollar has appreciated (i.e. the exchange rate has fallen), the importer will pay a higher price by buying pounds through the forward contract, but will have eliminated all uncertainty over the cost of the pounds (c) ECI Risk Training 2009
11 The advantage to hedging with a forward contract is that the contract can be customized to the needs of the hedger The counterparties can agree to any underlying asset and any delivery time (c) ECI Risk Training 2009
12 The disadvantage is that the hedger cannot benefit from changes in the risk factor being hedged For example, if an importer hedges against the risk of a depreciating dollar with a forward contract, he does not save if the dollar appreciates (c) ECI Risk Training 2009
13 FUTURES CONTRACTS A futures contract is similar to a forward contract; the main differences are: futures contracts are traded on organized exchanges, while forward contracts are traded in the over-the-counter market (c) ECI Risk Training 2009
14 futures contracts are not subject to counterparty risk, since the derivatives exchanges guarantee their performance; forward contracts are subject to default risk futures contracts can easily be unwound (offset), while forward contracts cannot (c) ECI Risk Training 2009
15 futures contracts are “marked-to-market”; this requires an initial deposit in a margin account, and may require further funding throughout the lifetime of the contract forward contracts are not marked-to- market (c) ECI Risk Training 2009
16 SWAPS A swap is an agreement between two counterparties to exchange cash flows at regular intervals based on the difference between two variables, such as interest rates or exchange rates (c) ECI Risk Training 2009
17 EXAMPLE Suppose a firm has issued floating-rate debt that pays LIBOR (the London Interbank Offer Rate) to bondholders and wishes to hedge the risk that interest rates will rise in the future (c) ECI Risk Training 2009
18 The firm can enter into an interest rate swap with a swap dealer in which it agrees that every six months, it will: receive LIBOR from the dealer pay a fixed rate of interest to the dealer (c) ECI Risk Training 2009
19 This arrangement has the effect of transforming the firm’s floating-rate liability into a fixed-rate liability Suppose that the fixed rate is 5%; the relevant cash flows are shown in the following diagram: (c) ECI Risk Training 2009
20 (c) ECI Risk Training 2009
21 This diagram shows that the firm will use the LIBOR payments from the swap dealer to pay its bondholders; the 5% that the firm pays to the swap dealer now represents its interest rate costs (c) ECI Risk Training 2009
22 The firm now pays 5% each year instead of an unknown floating rate of interest (c) ECI Risk Training 2009
23 OPTIONS An option gives the buyer the right, but not the obligation, to buy or sell an asset in the future at a fixed price; the fixed price is known as the strike price or exercise price Options are traded on exchanges and in the over-the-counter market (c) ECI Risk Training 2009
24 OPTIONS CLASSIFICATION Call option: provides the right to buy an asset Put option: provides the right to sell an asset (c) ECI Risk Training 2009
25 European option: can be exercised only on its maturity date American option: can be exercised at any time prior to maturity (c) ECI Risk Training 2009
THE BASIC PROPERTIES 26 OF CALL OPTIONS The payoff to a call option is: MAX (S-X, 0) (c) ECI Risk Training 2009
27 where: MAX = maximum S = price of underlying asset X = strike (exercise) price (c) ECI Risk Training 2009
28 How is this payoff determined? Suppose that an investor owns a call option with strike price X. On the option’s maturity date, the market price of the underlying asset is S. (c) ECI Risk Training 2009
29 If S exceeds X, the option will be exercised; the investor will be able to buy the asset at a price of X and resell it at a price of S, for a payoff of S – X If S is less than or equal to X, the investor will not exercise the option; its payoff is therefore 0 (c) ECI Risk Training 2009
30 In the first scenario, the payoff is S-X, which is a positive number. In the second scenario, the payoff is 0. Therefore, the payoff is the greater of S-X and 0, which is expressed as: MAX(S – X, 0) (c) ECI Risk Training 2009
31 EXAMPLE (c) ECI Risk Training 2009
32 PAYOFF DIAGRAM The payoffs to this option are shown in the following table and diagram: (c) ECI Risk Training 2009
S X Payoff = 33 MAX(S-X,0) 30 50 0 35 50 0 40 50 0 45 50 0 50 50 0 55 50 5 60 50 10 65 50 15 70 50 20 75 50 25 (c) ECI Risk Training 2009
Payoff to IBM Call Option 34 30 25 20 Payoff ($) 15 10 5 0 30 35 40 45 50 55 60 65 70 75 Stock Price ($) (c) ECI Risk Training 2009
35 PROFIT/LOSS An option will be exercised as long as the payoff is positive. Whether the investor earns a profit or loss depends on the price paid for the option. (c) ECI Risk Training 2009
36 For the owner of the call option, the profit or loss will be: PAYOFF – PRICE = MAX(S – X,0) – C where: C = call price (c) ECI Risk Training 2009
37 PROFIT/LOSS DIAGRAMS The profits and losses to this option are shown in the following table and diagram: (c) ECI Risk Training 2009
S X Payoff = C Profit = 38 MAX(S-X,0) MAX(S-X,0) – C 30 50 0 3 -3 35 50 0 3 -3 40 50 0 3 -3 45 50 0 3 -3 50 50 0 3 -3 55 50 5 3 2 60 50 10 3 7 65 50 15 3 12 70 50 20 3 17 75 50 25 3 22 (c) ECI Risk Training 2009
Profit/Loss to IBM Call Option 39 25 20 15 Profit/Loss ($) 10 5 0 30 35 40 45 50 55 60 65 70 75 -5 Stock Price ($) (c) ECI Risk Training 2009
40 NOTE The maximum loss per share equals the call price of $3; this occurs for all S ≤ X (c) ECI Risk Training 2009
41 For all S > X, the investor’s profit per share increases by $1 for each $1 increase in S; therefore, there is no maximum profit (c) ECI Risk Training 2009
42 TERMINOLOGY When S > X, a call is in the money When S = X, a call is at the money When S < X, a call is out of the money (c) ECI Risk Training 2009
43 THE BASIC PROPERTIES OF PUT OPTIONS The payoff to a put option is defined as: MAX (X — S, 0) (c) ECI Risk Training 2009
44 where: MAX = maximum S = price of underlying asset X = strike (exercise) price (c) ECI Risk Training 2009
45 How is this payoff determined? Suppose that an investor owns a put option with strike price X. On the option’s maturity date, the market price of the underlying asset is S. (c) ECI Risk Training 2009
46 If S is less than or equal to X, the option will be exercised; the investor will be able to buy the asset at a price of S and sell the asset at a price of X, for a payoff of X – S If S exceeds X, the option will be not exercised; its payoff is therefore 0 (c) ECI Risk Training 2009
47 In the first scenario, the payoff is X — S, which is a positive number. In the second scenario, the payoff is 0. Therefore, the payoff is the greater of X-S and 0, which is expressed as: MAX(X – S, 0) (c) ECI Risk Training 2009
48 (c) ECI Risk Training 2009
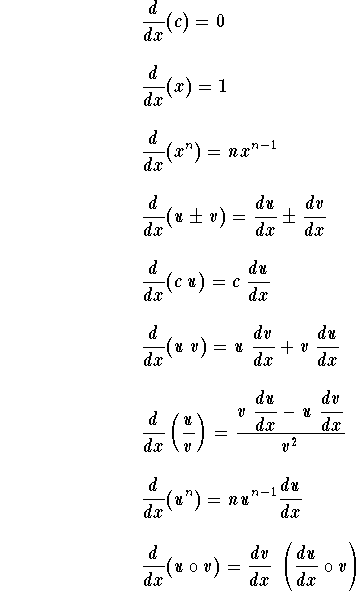
49 PAYOFF DIAGRAM The payoffs to this option are shown in the following table and diagram: (c) ECI Risk Training 2009
S X Payoff = 50 MAX(X-S,0) 30 50 20 35 50 15 40 50 10 45 50 5 50 50 0 55 50 0 60 50 0 65 50 0 70 50 0 75 50 0 (c) ECI Risk Training 2009
Payoff to IBM Put Option 51 25 20 15 Payoff ($) 10 5 0 30 35 40 45 50 55 60 65 70 75 Stock Price ($) (c) ECI Risk Training 2009
52 PROFIT/LOSS An option will be exercised as long as the payoff is positive. Whether the investor earns a profit or loss depends on the price paid for the option. (c) ECI Risk Training 2009
53 For the owner of the put option, the profit or loss will be: PAYOFF – PRICE = MAX(X – S,0) – P where: P = put price (c) ECI Risk Training 2009
54 PROFIT/LOSS DIAGRAMS The profits/losses to this option are shown in the following table and diagram: (c) ECI Risk Training 2009
S X Payoff = P Profit = 55 MAX(X-S,0) MAX(X-S,0) – P 30 50 20 2 18 35 50 15 2 13 40 50 10 2 8 45 50 5 2 3 50 50 0 2 -2 55 50 0 2 -2 60 50 0 2 -2 65 50 0 2 -2 70 50 0 2 -2 75 50 0 2 -2 (c) ECI Risk Training 2009
Profit/Loss to IBM Put Option 56 20 15 10 Profit/Loss ($) 5 0 30 35 40 45 50 55 60 65 70 75 -5 Stock Price ($) (c) ECI Risk Training 2009
57 NOTE The maximum loss per share equals the put premium of $2; this occurs for all S ≥ X (c) ECI Risk Training 2009
58 For all S < X, the investor’s profit per share increases by $1 for each $1 decrease in S; profit reaches a maximum of $X only in the unlikely event that S falls to zero (c) ECI Risk Training 2009
59 TERMINOLOGY When S < X, a put is in the money When S = X, a put is at the money When S > X, a put is out of the money (c) ECI Risk Training 2009
60 HEDGING WITH OPTIONS If a hedger needs to buy an asset in the future, the risk of rising future prices can be hedged with a call option If the price of the underlying asset rises above the option’s strike price, the hedger can buy the asset at the strike price; if not, the hedger can buy the asset at the lower market price (c) ECI Risk Training 2009
61 If a hedger needs to sell an asset in the future, the risk of falling future prices can be hedged with a put option If the price of the underlying asset falls below the option’s strike price, the hedger can sell the asset at the strike price; if not, the hedger can sell the asset at the higher market price (c) ECI Risk Training 2009
62 EXAMPLE Suppose that a jewelry manufacturer needs to buy silver in six months. The manufacturer faces the risk that the price of silver will rise in six months. In order to hedge this risk, the manufacturer buys a call option on silver. Assume that the strike price of the option is $5/ounce. (c) ECI Risk Training 2009
63 In six months, there are two possible scenarios: 1) the market price is greater than $5 In this case, the manufacturer exercises the option and buys silver at $5 (c) ECI Risk Training 2009
64 2) the market price is less than or equal to $5 In this case, the manufacturer does not exercise the option and buys silver at the market price (c) ECI Risk Training 2009
65 The manufacturer has set a limit on the price that it will pay for silver in six months ($5) while retaining the ability to pay a lower price (c) ECI Risk Training 2009
66 EXAMPLE Suppose that an oil company will sell crude oil in three months. The oil company faces the risk that the price of oil will fall in three months. In order to hedge this risk, the oil company buys a put option on oil. Assume that the strike price of the option is $50/barrel. (c) ECI Risk Training 2009
67 In three months, there are two possible scenarios: 1) the market price is greater than $50 In this case, the oil company does not exercise the option and sells oil at the market price (c) ECI Risk Training 2009
68 2) the market price is less than or equal to $50 In this case, the oil company exercises the option and sells oil at the strike price of $50 (c) ECI Risk Training 2009
69 The oil has set a minimum price at which it will be able to sell its oil ($50) while retaining the ability to sell the oil at a higher price (c) ECI Risk Training 2009
70 HEDGING WITH FORWARD CONTRACTS VS. OPTIONS The advantage to hedging with forward contracts is that there is no cost to the hedger The disadvantage is that the hedger locks in a specific price, and cannot benefit from favorable movements in market prices (c) ECI Risk Training 2009
71 The advantage to hedging with options is the ability to benefit from favorable market movements in market prices while guaranteeing a maximum price at which an asset can be bought or a minimum price at which it can be sold (c) ECI Risk Training 2009
72 The disadvantage to hedging with options is that the hedger must pay a price to buy an option (c) ECI Risk Training 2009














