Instructor: Jay Wagner

Learn about the Capital Asset Pricing Model (CAPM), one of the foundational models in finance. We’ll look at the underlying assumptions, how the model is calculated, and what it can do for you.

The Capital Asset Pricing Model — Definition and Underlying Assumptions

In finance, one of the most important things to remember is that return is a function of risk. This means that the more risk you take, the higher your potential return should be to offset your increased chance for loss.

One tool that finance professionals use to calculate the return that an investment should bring is the Capital Asset Pricing Model (CAPM from now on). CAPM calculates a required return based on a risk measurement. To do this, the model relies on a risk multiplier called the beta coefficient, which we will discuss in the next section.

Like all financial models, the CAPM depends on certain assumptions. Originally, there were nine assumptions, although more recent work in financial theory has relaxed these rules somewhat. The original assumptions were:

1. Investors are wealth maximizers who select investments based on expected return and standard deviation.
2. Investors can borrow or lend unlimited amounts at a risk-free (or zero risk) rate.
3. There are no restrictions on short sales (selling securities that you don’t yet own) of any financial asset.
4. All investors have the same expectations related to the market.
5. All financial assets are fully divisible (you can buy and sell as much or as little as you like) and can be sold at any time at the market price.
6. There are no transaction costs.

7. There are no taxes.
8. No investor’s activities can influence market prices.
9. The quantities of all financial assets are given and fixed.

Obviously, some of these assumptions are not valid in the real world (most notably numbers 6 and 7), but CAPM still works well, and results can be adjusted to overcome some of these assumptions.

The Beta Coefficient

Before we can use the CAPM formula, we need to understand its risk measurement factor, the beta coefficient. By definition, the securities market as a whole has a beta coefficient of 1.0. The beta coefficients of individual companies are calculated (by the investment research firm Value Line, among others) relative to the market’s beta. A beta above 1.0 implies a higher risk than the market average, and a beta below 1.0 implies less risk than the market average. Most companies’ betas fall between 0.75 and 1.50, but any number is possible, including negative numbers (a negative beta would be highly unlikely, however, since it would imply less risk than a ‘risk free’ investment).

For actual use, the beta coefficients of most companies can be found on financial websites such as Yahoo Finance and Bloomberg, as well as in Value Line’s paper and electronic publications. A quick sample of five randomly selected companies (using Yahoo Finance) showed the following beta coefficients as of 2014:

• Apple: 0.74
• Berkshire Hathaway: 0.25
• Ford Motor Company: 1.34
• Microsoft: 0.68
• Sony Corporation: 1.82

We will use a few of these later to demonstrate the use of the CAPM.

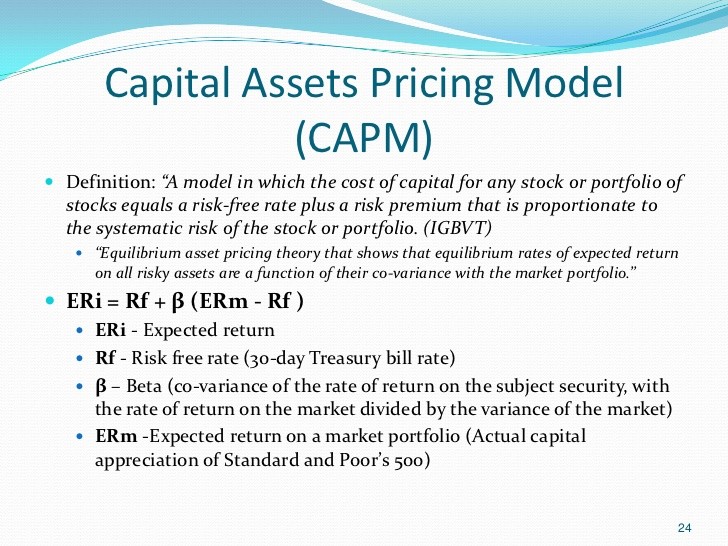
The CAPM Formula and Examples

The CAPM formula is sometimes called the Security Market Line formula and consists of the following equation:

r* = kRF + b(kM — kRF)

It is basically the equation of a line, where:

r* = required return

kRF = the risk-free rate

kM = the average market return