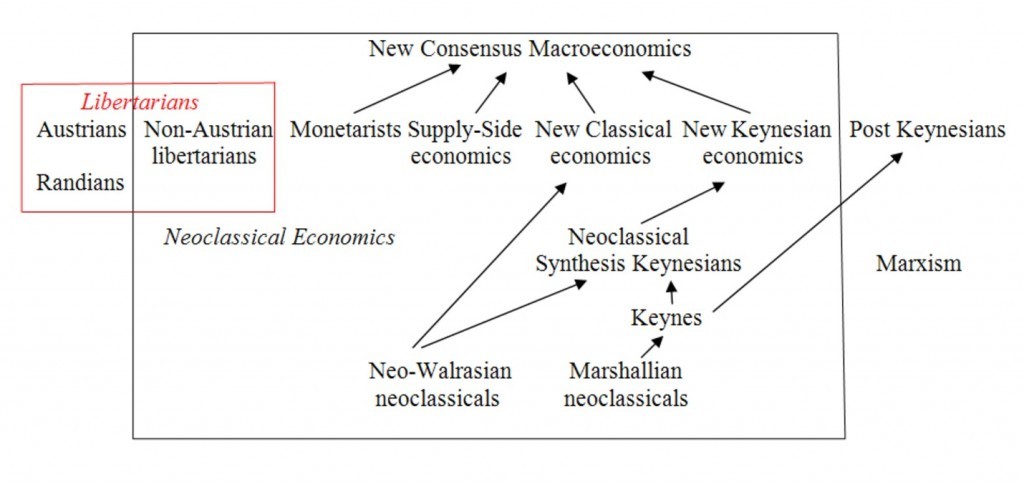
Neoclassical economic theory

Post on: 30 Апрель, 2015 No Comment

www.jstor.org/stable/2222645, via Wikimedia Commons)

Origins of Neoclassical Economic Theory

In textbooks on economics, the creators of neoclassical economics (Stanley Jevons. Leon Walras, Francis Ysidro Edgeworth, and Vilfredo Pareto) are credited with transforming the study of economics into a rigorously mathematical scientific discipline. There are, however, no mentions in these textbooks, or in all but a few books on the history of economic thought, of a rather salient fact. The progenitors of neoclassical economics, all of whom were trained as engineers, developed their theories by substituting economic constructs derived from classical economics for physical variables in the equations of mid-nineteenth century theory in physics.

The physics that the creators of neoclassical economics used as the template for their theories was developed from the 1840s to the 1860s. During this period, physicists responded to the inability of Newtonian mechanics to account for the phenomena of heat. light, and electricity with a profusion of hypotheses about matter and forces. In 1847 Hermann-Ludwig Ferdinand von Helmholtz. one of the best known and most widely respected physicists at this time, posited the existence of a vague and ill-defined energy that could unify these phenomena. This served as a catalyst for a movement in which physicists attempted to explain very diverse physical phenomena in terms of a unified and protean field of energy.

Hermann von Helmholtz.

Because the physicists were unable to specify the actual character of this energy and could not be precise about what was being measured, their theories were not subject to repeatable experiments under controlled conditions. Obviously, this violated one of the cardinal rules of the scientific methodthe predictions of any scientific theory must be testable and potentially falsifiable in repeatable experiments under controlled conditions. The amorphous character of energy in the physical theories also obliged the physicists to appeal to the law of the conservation of energy. This appeal was necessary because it was the only means of asserting that the vaguely defined system somehow remains the same as it undergoes changes and transformations.

The creators of neoclassical economics began with the assumption that a particle or mass point could be viewed as the equivalent of an atomized economic actor that moves along a path in accordance with the principle of least action. Aware that energy in the equations of mid-nineteenth century physics is a force that pervades all space, the economists concluded that this space could also be filled by a postulated form of energy called utility. None of these figures appears to have seriously considered the fact that utility, defined as economic satisfaction and well being, cannot be directly known or measured and is in no way comparable to energy as that term was used in mid-nineteenth century physics. Equally remarkable, they also dismissed or rationalized away issues of integration and invariance that are critically important in the proper application of the conservation principle.

The strategy used by the economists was remarkably simplethey took the equations from the mid-nineteenth century physical theory and changed the names of the variables. Utility was substituted for energy. the sum of utility for potential energy, and expenditure for kinetic energy. Although there was no basis for claiming that the natural laws of economics are in any sense the equivalent of the physical variables, the strategy allowed utility to be treated as a field of vector potentials in which the sum of income and utility is conserved. None of these now famous people seemed to realize that the sum of income and utility in neoclassical economics, much less in economic reality, is not conserved and that the conservation principle is quite meaningless in any real economic process. Nevertheless, this blatantly unscientific assumption is now used to legitimate the existence of the invisible hand in its current formconstrained maximization in general equilibrium theory.

Circular flow in closed market system.

In the mathematical formalism that resulted from these substitutions, the mind of atomized economic actors is presumed to operate within a field of force identified, in both figurative and literal terms, with energy. The forces associated with this energy were represented as prices and spatial coordinates described quantities of goods. Because utility-energy in this formalism is conserved, the creators of neoclassical economic theory were obliged to view production and consumption of goods and commodities as physically neutral processes that do not alter the sum of utility. They did so by arriving at a very strange interpretation of the now outmoded law of the conservation of matter, or the idea that matter cannot be created or destroyed. If matter, they argued, is immutable, then the production of goods and commodities cannot alter or change the stuff out of which goods or commodities are made. They then claimed that any value which accrues as a result of production can only reside in the mental space of economic actors. In an effort to justify this conclusion, the economists argued that if the immutable stuff out of which goods or commodities are made cannot be changed by consumption, any value associated with consumption must reside in the minds of economic actors.

This strange view of substance in economic reality was used to interpret the meaning of the economic variables in the equations borrowed from mid-nineteenth century physics and this served as part of the rationale for the theory of value in neoclassical economic theory. In the formalism of this theory, the atomized immaterial minds of economic actors operate within a field of force (utility) in which the natural laws of economics are presumed to legislate over the choices made by the actors. This became the basis for a fundamental assumption in neoclassical economic paradigmthe value assigned to all the immutable unchanging stuff that circulates in a closed loop from production to consumption in a market system results from the operation of the putative natural laws.

The Creators of Neoclassical Economics

William Stanley Jevons.

William Stanley Jevons. after being encouraged by his father to become an engineer, studied chemistry and mathematics in London and attended some of Michael Faradays lectures at the Royal Institution. In these lectures, Faraday demonstrated that magnetic forces did not obey the Newtonian force rule and argued that other forces must be present. Jevons was also familiar with the work of Thompson and Joule on the inter-convertibility of heat and mechanical energy that laid the foundations for the law of the conservation of energy. But since Jevons was not a skilled mathematician, his understanding of scientific matters was crude at best and completely distorted at worst.

In order to appreciate why Jevons appeared to have no difficulty identifying mind or consciousness in economic reality with a point particle moving in a field of energy. consider the following passage from his major work, The Principles of Science :

Life seems to be nothing but a special form of energy which is manifested in heat and electricity and mechanical force. The time may come, it almost seems, when the tender mechanism of the brain will be traced out, and every thought reduced to the expenditure of a determinate weight of nitrogen and phosphorous. No apparent limit exists to the success of the scientific method in weighing and measuring, and reducing beneath the sway of law, the phenomena of matter and mindMust not the same inexorable reign of law which is apparent in the motions of brute matter be extended to the human heart?

Mind, says Jevons, is a manifestation of energy. the physical substrate of mind can be reduced to a measurable quantity, such as the weight of nitrogen and phosphorous, and the phenomena of mind are potentially explainable in terms of collections of particles subject to the inexorable reign of deterministic physical laws. If one actually believed, as Jevons apparently did, that this is the case, it would not require a great leap of faith to arrive at what is, in retrospect, a very strange conclusion. If mind in economic reality is a manifestation of energy that is similar to or the same as the protean field of amorphous energy described in mid-nineteenth century physics, utility can be substituted for energy in the equations of this physics.

In spite of the fact that Jevons was quite convinced that his appropriation of the equations of mid-nineteenth century physics had transformed economics into a science, his understanding of the ontological status of the natural laws that allegedly act between or outside the parts (economic actors) to maintain the stability of the whole (market system) was the same as that of Adam Smith. In the following passage from Theory of Political Economy. Jevons defends the claim that his theory is scientific:

It is clear that Economics, if it is to be a science at all, must be a mathematical science. There exists much prejudice against attempts to introduce the methods and language of mathematics into any branch of the moral sciences. Many people seem to think that the physical sciences form the proper sphere of mathematical method, and that the moral sciences demand some other methodI know not what. My theory of Economics, however, is purely mathematical in character. Nay, believing that the quantities with which we deal must be subject to continuous variation, I do not hesitate to use the appropriate branch of mathematical science, involving though it does the fearless consideration of infinitely small quantities. The theory consists of applying differential calculus to the familiar notions of wealth, utility, value. demand, supply, capital. interest, labour, and all the other quantitative notions belonging to the daily operations of industry.To me it seems that our science must be mathematical, simply because it deals in quantities. Whenever things treated are capable of being greater or less, there the laws and relations must be mathematical in nature.

Jevons argues that because his theory deals in quantities that are subject to continuous variation, this justifies the translation of nebulous constructs in economic theory into well-defined quantities with the use of the differential calculus. In classical physics, differential calculus is used to describe the movement of point particles in vector space in terms of continuous functions that result in infinitely small differentials in accordance with the classical laws of motion. Jevons seems quite oblivious to the fact that economic actors cannot be described in this fashion and one does not have to be a trained logician to appreciate that his argument is circularthe theory must be scientific because it is mathematical and the theory must be mathematical because it is scientific. This same argument would be used repeatedly by subsequent generations of mainstream to defend the claim that economics is a rigorously mathematical discipline comparable to physics.

Leon Walras, who was also encouraged by his father to study engineering, enrolled in the Ecole des Mines in 1845. Dissatisfied with the study of engineering, Walras read philosophy, history, literary criticism, and political economy. During this period, he also read a popular account of the philosophy of Kant and embraced a confused monism that was a synthesis of materialism and spiritualism. In spite of his lack of training in either mathematics or physics, Walras viewed Newtonian astronomy and classical mechanics as the unequaled models of scientific knowledge and his grand ambition was to use these models to create the science of economic forces, analogous to the science of astronomical forces.

Like Jevons, Walras posited an additive utility function in which the utility of a good is solely the function of the quantity of the good consumed. The additive utility function allowed the utility of a bundle of goods to be expressed as the sum of the single utility functions that allegedly expresses the pleasure derived by the consumer in his or her consumption of each good in the bundle. Walras rarete, which is the equivalent to Jevons marginal utility, refers to the last increment of utility (pleasure) derived by a consumer from an infinitesimal increment in the consumption of a particular good. He also claimed that while the marginal utility of a good is positive, the added utility for the consumer of successive amounts of a particular good gradually diminishes. For example, the first piece of bread consumed by a hungry man would have the most added utility and the amount of utility associated with consuming subsequent pieces would gradually diminish.

In Elements of Pure Economics. Walras makes a stark distinction between forces associated with the natural laws of economics and the force of free will:

We may divide the facts of our universe into two categories: those which result from the play of blind and ineluctable forces of nature and those which result from the exercise of free will, a force that is free and cognitive. Facts of the first category are found in nature, and that is why we call them natural phenomena. Facts of the second category are found in man, and that is why we call them human phenomena..The operations of the forces of nature constitute the subject matter of what is called pure natural science or science properly speaking. The operation of the human will constitute, in the first place, the subject matter of what is called pure moral science or history, and in the second place, as will be seen presently, the subject matter of a study to which another name, either art or ethics, is attached.

Walras claims that all natural forces that operate outside the atomized human mind are blind and ineluctable and he includes in these forces those associated with the natural laws of economics. Later in this same discussion, the distinction between the natural and the human becomes a distinction between things and persons, and this distinction becomes the basis for yet another distinction between the relations of persons and things in industry and the relations between persons and other persons in institutions. Walras then concludes, The theory of industry is called applied science or art; the theory of institutions moral science or ethics.

This categorical distinction between the domain of economics and all other human domains, including government, became one of the central dogmas of mainstream economics. But how does Walras justify its existence? He does so by claiming that there is only one natural phenomenon in economic realitythe single relation between two things represented by the value or price of a good. Thus, he argues, any value in exchange, once established, partakes of the character of a natural phenomenon, natural in origins, natural in its manifestations and natural in essence.

Assuming that natural means from or pertaining to nature, on what basis does Walras conclude that a value established in an exchange can be viewed as natural in its origins, manifestations and essence? Markets are human inventions that have taken a wide range of different forms, the value of any commodity is normally a function of a staggering array of variables, and prices paid are invariably tied to individual tastes and preferences. For Walras, however, none of this matters because he assumes that prices are governed by deterministic natural laws, and this allows him to argue that these prices are natural in origins, manifestations and essence. When contemporary mainstream economists use the term natural, as in natural rates of unemployment. they rarely comment on its meaning. Their use of the word implies, however, that there is a natural lawful order in market systems and that whatever is natural must be good. And this serves to reinforce the view that outside intervention by government or other agency on closed market systems will disrupt the otherwise inevitable progress toward the good.

Although Walras natural economic order is very rigid and highly mechanistic, he does not claim that human will has no influence on prices. But he does say that the forces that regulate comparative prices are comparable to the law of gravity, and this becomes the basis for the following argument. Just as the force of human will can resist the force of gravity, it can also resist the forces that regulate competitive prices. Yet one cannot, says Walras, fundamentally alter the manner in which economic forces govern the interaction of atomized economic actors any more that one can alter the manner in which gravity governs the interactions of point particles. Because the force of gravity in classical physics tends to move physical systems toward equilibrium, Walras concludes that economic forces tend to move competitive prices toward equilibrium. It is this more restricted view of determinism that allegedly legitimates his theory of the determination of prices under a hypothetical regime of perfect competition.

The fact that the neoclassical model in mainstream economics was derived from this incredibly inept manipulation of a soon-to-be outmoded physical theory would soon be forgotten. What would survive, however, is the assumption that the usefulness of economic models in neoclassical economics is a form of scientific proof. Note how Walras exploits this idea in an attempt to reinforce his claim that economics is a science in the following commentary on the relationship between geometry and prices:

Force and velocity are also measurable magnitudes, but the mathematical theory of force and velocity is not the whole of mechanics. Nevertheless, pure mechanics surely ought to precede applied mechanics. Similarly, given the pure theory of economics, it must be applied economics; and this pure theory of economics is a science which resembles the physico-mathematical sciences in every respect. This assertion is new and will seem strange; but I have proven it to be true, and I shall elaborate the proof in what follows.

If the pure theory of economics or the theory of exchange and value in exchange, that is, the theory of social wealth considered by itself, is a physico-mathematical science like mechanics or hydrodynamics, then economists should not be afraid to use the methods and language of mathematics.

Walras argues that since pure mathematics precedes applied science, it follows that pure economics should precede applied economics. The only basis for the claim that his economics is pure is that it represents vague economic concepts in the mathematical equations of mid-nineteenth century physics. Yet this is the basis for his proof that the resulting economic theory resembles the physico-mathematical sciences in every respect and should be viewed as a science like mechanics or hydrodynamics.

In the cryptic discussions of the origins of neoclassical economic theory found in most introductory economics textbooks, the claim is normally made that this theory was independently and simultaneously discovered in the 1870s by the Englishman Jevons. the Frenchman Walras, and the Austrian Carl Menger (1840-1921). The implication is that any theory that emerges independently and simultaneously in very different cultural contexts must be empirically valid. As the institutional economist Philip Mirowski has demonstrated in exhaustive detail, however, this discovery was not simultaneous and the inclusion of Menger in the pantheon of the first neoclassical economics was an historical accident.

Menger did claim that he was one of the originators of neoclassical economic theory, but he rejected the unifying principle of the theory, the construct of utility, and made no use of the new mathematical techniques borrowed from mid-nineteenth century physics. Mirowski agues that the primary reason why Mengers false claim was taken seriously is that one of his illustrious students, Friedrich von Wieser, managed to successfully promote it during a period in which the work of his former teacher was largely unavailable outside of the German speaking world. In any event, the argument that general equilibrium theory must reveal truths about economic reality because of the manner in which it was discovered is bogus.

Francis Ysidro Edgeworth and Vilfredo Pareto embraced Walras claim that utility was the equivalent of energy in the equations of mid-nineteenth century physics along with his conclusion that economics had become a rigorously scientific discipline. The following is Edgeworths commentary on this subject:

The application of mathematics to the world of the soul is countenanced by the hypothesis (agreeable to the general hypothesis that every psychical phenomena is the concomitant, and in some the sense the other side of a physical phenomena), the particular hypothesis, adopted in these pages, that Pleasure is the concomitant of Energy. Energy may be regarded as the central idea of Mathematical Physics: maximum energy the object of the principle investigations in that scienceMechanique Sociale may one day take her place along with Mechanique Celeste, throned each upon the double-sided height of one maximal principle, the supreme principle of moral as of physical science. As the movements of each particle, constrained or loose, in a material cosmos are continually subjugated to one maximum sub-total of accumulated energy. so the movements of each soul whether selfishly isolated or linked sympathetically, may continually be realizing the maximum of pleasure.

Paretos position, although more pugnacious, is essentially the same as that of Edgeworth:

Strange disputes about predestination, about the efficacy of grace, etc. and in our own day incoherent ramblings on solidarity show that men have not freed themselves from these daydreams which have been gotten rid of in the physical sciences, but which still burden the social sciencesThanks to the use of mathematics, this entire theory, as we develop it in the Appendix, rests on no more than a fact of experience, that is, on the determination of the quantities of goods which constitute combinations between which the individual is indifferent. The theory of economic science thus acquires the rigor of rational mechanics.

More Recent Developments in Neoclassical Economics

Alfred Marshall.

Alfred Marshal, the dominant figure in mainstream economics from 1890 to the beginning of World War I, popularized neoclassical economic theory and altered some of the work he promoted. Many historians of economics have cited Marshalls claim that the Mecca of the economist lies in economic biology and concluded that he preferred the biological metaphor to the physics metaphor. In the next sentence, however, Marshall writes, But biological conceptions are more complex that those of mechanics; a volume on Foundations must therefore give a relatively large place to mechanical analogies Similarly, those who frequently quote Marshalls pronouncement burn the mathematics should read the following passage from the eighth edition of his Principles of Economics :

The new analysis is endeavoring gradually and tentatively to bring over into economics, as far as the widely different nature of the material allow, those methods of the science of small increments (commonly called the differential calculus) to which man owes directly or indirectly the greater part of the control that he has obtained in recent times over physical nature. It is still in its infancy; it has no dogmas, and there is no standard orthodoxyThere is a remarkable harmony and agreement on essentials among those who are working constructively by the new method; and especially among such of them as have served an apprenticeship in the simpler and more definite, and therefore more advanced, problems of physics.

When Marshall made this comment, the physical theory used by the creators of neoclassical economics was outmoded, but he seems to be completely unaware that this was the case.

John Maynard Keynes.

John Maynard Keynes, a student of Marshall, wrote his most seminal work during a period in which the Great Depression was challenging neoclassical assumptions about the lawful mechanisms of market systems. In 1935, Keynes said the following in a letter to playwright George Bernard Shaw: you have to know that I am writing a book on economic theory which will largely revolutionizenot as I suppose at once, but in the course of the next ten yearsthe way the world thinks about economic problems. What was most radical about the book that Keynes felt would occasion this revolution, The General Theory of Employment, Interest and Money. is that it grounds economic processes in historical time, or in a more experiential sense of time where the future cannot be known and the past cannot be changed.

The mathematical formalism borrowed from the equations of the mid-nineteenth century theory in physics obliged Walras to view economic actors as imbued with prodigious knowledge of economic variables and existing in a wholly abstract realm where time in all of its real or actual dimensions does not exist. Keynes more recognizably human economic actor is quite different. He or she is motivated in part by animal spirits and irrational desires and inhabits an economic reality in which knowledge is always proximate and future outcomes are essentially indeterminate.

In The General Theory. Keynes first makes the case that there is nothing inherent in the mechanisms of a free market system to prevent a situation in which surplus savings does not result in lower interest rates and investment spending plummets due to expectations of future low sales. In the absence of borrowing and investment spending, there is, he concluded, no economic impetus to expand or grow the economy. As the world-wide depression in the 1930s had shown, these conditions could lead to some very unfortunate resultsmassive unemployment. a spiral of contraction due to lack of spending on capital equipment, and a climate of uncertainty in which private investment was not sufficient to reverse the economic decline. Keynes well-known solution to this problem is that government should take up the slack by funding projects that employ the unemployed. The monies earned by these individuals would, said Keynes, increase the buying power that fuels consumption and lead to resumption of private investment and business expansion.

There was much that was troubling, then and now, about The General Theory from the perspective of neoclassical economists. Keynes claim that the unimpeded operations of the natural laws of economics can result in a situation in which an economy not only fails to grow but even contracts suggested that the laws of economics were fallible. Because the prescribed remedy for this situation was large-scale intervention by government, or by an agency outside the closed market system, this not only suggested that the system cannot under all conditions be viewed as closed. It also indicated that there were situations where the natural laws of economics, if left alone, could not sustain the economic well-being of even the majority of economic actors.

The realization that led Keynes to develop a theory that would later be dubbed the Keynesian heresy is apparent a letter he wrote in 1934 to economist John Hicks: I shall hope to convince you some day that Walrass theory and all the others along these lines are little better than nonsense. If Keynes had been able to convince other neoclassical economists that Walrasian general equilibrium theory is nonsense, then the revolution he had in mind might have actually occurred. This did not happen, however, because Keynes wed new assumptions about economic reality to a mathematical formalism that was essentially the same as that of Walras.

Keynes suggestion that the behavior of economic actors and firms cannot under certain conditions prevent the market system from moving toward a state of general equilibrium resulted in the development of macroeconomics. Based largely on the broadly homogenous categories of economic activity developed by Keynes, macroeconomists attempt to study the whole of the economy by representing the economic behavior of economic actors and firms within these categories as lawfully determined based on deductions from general equilibrium theory. The resulting mathematical models are used to assess macroeconomic issues, such as the effects of government policies on inflation and unemployment. the impacts of changes in the overnight interest rates by the central bank on stock markets, and the overall costs associated with increases in the minimum wage. Virtually all of the macroeconomic models are extensions of the means and methods of the microeconomic models in general equilibrium theory and predicated on the same assumptions about the allegedly lawful dynamics of market systems.

Jon von Neumann.

In the 1930s and 1940s, the foundations were laid for the axiomatization of general equilibrium theory, or for reformulating this theory within a framework of hypotheses perfectly delineated and rigorously expressed in mathematical language. The impulse toward axiomatization came from two gifted mathematicians, John von Neumann and Oskar Morgenstern, who were highly critical of the conversational and imprecise language used by neoclassical economists. In conversation with Morgenstern, von Neumann said the following about the state of research in this discipline at the end of the 1930s:

You know, Oskar, if those books are unearthed sometime a hundred years hence, people will not believe they were written in our time. Rather they will think that they are contemporary with Newton. so primitive is their mathematics. Economics is simply still a million miles away from the state in which an advanced science is, such as physics.

Morgenstern was similarly disdainful of the state of economic theory. In a review of John Hicks Value and Capital (1939), Morgenstern dismissed the value of this well received and widely read attempt to develop a more comprehensive economic theory based on Walrasian analysis by concluding that it was outdated and lacking in rigor.

The rigor that von Neumann and Morgenstern wished to introduce into economic theory was predicated on the belief, popular in intellectual circles at the time, that more sophisticated mathematical techniques could disclose the underlying dynamics of human consciousness and decision-making. As Morgenstern put it,

It suffices to be familiar with the present state of the investigations of the foundations of mathematics to realize that all mathematical theorems are derived without exception from logic and solely from logic. From this follows that the potential use of mathematics in the social sciences means nothing else but that their problems can be formulated and treated in an exact manner.

The notion that timeless, universal truths govern the dynamics of human thought and behavior and that the essence of these truths consists of immaterial ideas, or preexisting logics, that can be uncovered by advances in mathematical theory is everywhere present in the work of von Neumann and Morgenstern. Both figures may have been very much aware that there was no basis for claiming that the mathematical theories developed by mainstream economists in the 1930s were scientific. But they also believed that a scientific theory could be developed with the use of higher mathematics.

One irony here is that their attempts to create this scientific theory served to perpetuate the fiction that the invisible hand actually exists by disguising the metaphysical assumptions upon which its existence is predicated under increasingly elaborate layers of mathematical formalism. Another is that von Neumann and Morgenstern attempted to do so during a period in which their conception of the relationship between mathematical theory and physical reality was completely undermined by developments in quantum physics. This conception was also undermined in a somewhat different way by Godels demonstration in his incompleteness theorem that no finite system of mathematics can be used to derive all true mathematical statements and, therefore, no algorithm, or calculation procedure, can prove its own validity.

Most of the research in neoclassical economics since the 1940s, particularly in general equilibrium theory, has been based on extensions and refinements of the work of von Neumann and Morgenstern. Paul Samuelson in Foundations of Economic Analysis (1947) attempted to systematize general equilibrium theory with the use of organic methodologies and mathematical techniques that were less rigorous than those used by von Neumann and Morgenstern. This is, however, the first treatise in economic theory in which the formal mathematical apparatus is embedded in the main argument and not placed in appendices.

In Foundations. Samuelson assumes that any problem in economic theory can be reduced to a system of equations and that general equilibrium is the solution of all the equations that represent the lawful dynamics of a free market system. He defines a meaningful mathematical theorem as simply a hypothesis about empirical data which could conceivably be refuted, if only under ideal conditions. Samuelson then goes on to specify that a theorem may be indeterminate and practically difficult, or impossible to determineBut it is meaningful because under ideal circumstances an experiment could be devised whereby one could hope to refute the hypothesis.

The curious presumption that a system of idealized representations of economic activity in mathematical theorems could somehow be proven if an ideal experiment could somehow be conducted would soon become the primary justification for claim that neoclassical economic theory is scientific. However, the mathematical formalism in this theory is such that it is not possible to define the specific roles or behavior of economics actors based on the functional form of the equations. Hence hypotheses normally refer to some qualitative properties of the functions that allegedly emerge from the formalism, such as convexity or monotony.

Aware of this problem, Samuelson rationalizes away the fact that experimental verification of general equilibrium is impossible by claiming that this is an indispensable aspect of theoretical economics: It is precisely because theoretical economics does not confine itself to specific narrow types of functions that it is able to achieve wide generality in its initial formulation. If a scientist claimed that a scientific theory is useful because it is sufficiently general to disallow any prospect that its predictions can be subjected to experimental proof, he or she would be the laughing stock of the profession. Mainstream economists, however, have consistently used this argument to justify the claim that neoclassical economics is a science comparable to the physical sciences.

In the equations of physical theories, variables have a counterpart in physical reality that is observable or potentially observable and new physical theories are deemed valid only if they make predictions that can be confirmed in repeatable experiments under controlled conditions. The primary reason why the predictions of general equilibrium theory cannot be confirmed in this manner is rather obviousthe natural laws of economics exist only in the minds of those who believe in their existence. Another is that the variables in the mathematical formalism of this theory are self-referential functions that have no real or actual counterpart in physical reality. Given that these predictions are the product of a system of equations that refers only to itself, there is no basis in principle for confirming or denying them. This leads to the obvious conclusion that that there is simply no way in which to prove that the theory is valid or invalid in scientific terms.

Gerard Debreu, who sought to complete the work begun by von Neumann and Morgenstern, published a complete axiomatization of general equilibrium theory in Theory of Value (1959) in which the emphasis is shifted from the mathematical techniques of infinitesimal calculus to those of algebra and typology. He extends this mathematical apparatus to include a broad new range of quantitative and qualitative analysis with the aim of creating a formal structure that clearly reveals all the assumptions and the logical structure of analysis. This approach represents a major shift in neoclassical economic thought because it attempts to account for the formation of exchange values in pure mathematical language and without reference to the interpretive value of the concepts. As Debreu puts it, Allegiance to rigor dictates the axiomatic form of the analysis where the theory, in the strict sense, is logically disconnected from its interpretations.

The presumption behind this analysis is that a purely mathematical economic theory can uncover previously hidden lawful dynamics in economic reality just as purely mathematical theories in physics have uncovered hidden dynamics of physical reality. Given that the mathematical constructs in physics can be understood mathematically without any reference to their meaning in ordinary language, Debreu claims that this should also be the case for constructs in an axiomized mathematical treatment of general equilibrium theory. The problem with these assumptions is not merely that there is no one-to-one correspondence between the variables in this theory and the actual behavior of economic systems. It is also that the metaphysically-based constructs upon which the theory is constructed are the same as those in Walrasian general equilibrium theory and this fact is only thinly disguised by a mathematical formalism that differs in form and content from that used by Walras.

Debreu may have been successful in representing these constructs in a framework of hypotheses that are perfectly delineated and rigorously expressed in mathematical language. But this does not, in any sense, prove the validity of the assumptions and only serves to disguise their metaphysical foundations under another complex set of mathematical idealizations that say nothing in ordinary language about the actual character of the assumptions. Yet Debreu assumes that a self-referential mathematical system that has no real or actual counterpart in economic reality can disclose hidden lawful dynamics in this reality. For example, he claims that uncertainties about the future of market economies are due to the unknown choice that nature will make from the set of possible states in the world and that these uncertainties can be eliminated based on future extensions of axiomatization in neoclassical economic theory.

Another neoclassical economist with a talent for higher mathematics, Kenneth Arrow, collaborated with Debreu to develop the Arrow-Debreu model for general equilibrium theory (1954). This mathematical model describes a hypothetical perpetually expanding market system in which idealized economic actors possess an unlimited understanding of the consequences of their economic choices and engage in perfect competition without any transmission and information costs. In this model, general equilibrium theory is rigorously expressed in mathematical terms as a set of ideal conditions in an idealized economic system characterized by equilibrium of perfect competition. The mechanism in the model that allegedly confirms that these actors have made the mutually compatible decisions that result in this equilibrium is a set of signals, or market prices, which operates automatically even though the actors are totally unaware of its existence.

The history of general equilibrium theory is much more complex and detailed that this brief account suggests. But what is most important about this history in ecological terms is that the theory is now and always has been predicated on assumptions about the relationship between parts (economic actors and firms) and wholes (market systems) articulated by the creators of neoclassical economics. These assumptions were metaphysical in origins and assumed the guise of scientific truths after the creators of neoclassical economics incorporated them into a mathematical formalism borrowed from mid-nineteenth century physics. This myth was perpetuated in theories that disguised the metaphysical foundations of the assumptions under an increasingly more complex maze of mathematical formalism.

Game Theory

A fair number of economists over the past two decades, including luminaries like Arrow and Hand, have expressed doubt about the efficacy of general equilibrium theory, but it is still the central legitimating construct in mainstream economics. Within the community of mainstream economists, the most serious objections have been raised by proponents of game theory. Game theory in economics originated in 1944 with the publication of The Theory of Games and Economic Behavior by John von Neumann and Oskar Morgenstern. A game, explained the authors, is a set of rules and objectives and a ranking of objectives by a set of players. Assuming that the sets are discrete and well-defined, von Neumann and Morgenstern argued that they can be expressed in mathematical equations and manipulated by mathematical symbols to predict outcomes.

Martin Shubik.

Martin Shubik, perhaps the best known and most influential of the game theorists, has been consistently critical of Walrasian general equilibrium theory because it assumes that economic actors have no freedom to make mistakes or even to make choices about the economic process. He claims that an alternate and more realistic description of the behavior of economic actors can be disclosed in non-cooperative game theory. Non-cooperative game theory, writes Shubik, appears to be particularly useful for the study of mass phenomena in which the communication between individuals must be relatively low and individuals interact with a more or less faceless economy, polity or society.

These impersonal forces can be represented, says Shubik, through the construction of mathematical models in which the rules of the game derive not only from the economics and technology of the situation, but from the sociological and legal structure as well. In Shubiks mathematical models, the basic features of an economy (tastes, technologies and endowments) are essentially the same as those in conventional Walrasian models, and sociological, political and legal forces are represented mathematical as the rules that act arbitrarily on the closed economy from the outside. These rules do not, claims Shubik, lie within the sphere of the closed economy because they are not natural or do not operate in accordance the natural laws of economics. Although Shubik does not argue that the natural laws of economics are the single determinate of economic behavior, these laws function in his theory as the prime determinant of such behavior. The economic actor in his mathematical models may be buffeted by arbitrary variables that operate outside the closed market system, but this actor is still represented as a point particle subject to the influence of natural laws.

In a two-volume work entitled The Theory of Money and Financial Institutions. Shubik attempts to reconcile micro and macro economic theory by constructing a process-oriented theory of money and financial institutions based on a theory of games. He claims that it is possible to construct process models of the economy that are as rigorous as general equilibrium theory but explain more phenomena. Shubik then suggests that the essential problem with general equilibrium theory is that it is predicated on an absurdly reductive and restrictive view of economic actors: The rational, economic, institution-free individual assumed in its models is an overly simplistic abstraction of a subtle and complex creature who must function with high constraints on perception and ability to calculate, who uses both internal and external memory, and whose goals can hardly be well defined outside the context of the society in which the individual functions.

Shubik then notes that in the absence of well-defined models of politico-economic and socio-economic behavior, he is obliged to make the usual assumptions concerning the existence of well-defined preferences and utility functions, not because I believe in them, but because I want to show that even with these assumptions we can go beyond the results of general equilibrium theory. This effort to transcend the limits of general equilibrium theory is predicated on an interesting view of the institutions of society in general, and the financial institutions in particular. These institutions, writes Shubik, are the neural network of the sensors of the body economic, guiding the flows of funds, credits, and other financial paper that guide the real goods and services of the economy. He then proceeds to demonstrate that the process of setting up playable games forces us to invent minimal financial and governmental institutions even if we do not invoke the maximization of utility or use equilibrium as a general solution.

Based on the assumption that the neural network of financial institutions emerges as a logical necessity of decision making in market economies, Shubik claims that game theory can disclose the emergent logics that structures this network and this becomes the basis for two large claimsminimal financial institutions emerge as a logical, technological, and institutional necessity when economic activity is described as a playable game and both game theoretic and general-equilibrium models often predict the same apparently general, non-institutional outcome.

In the conclusion of this densely mathematical treatise, Shubix cautions that his game theoretic process models that can be solved for general equilibrium solutions are not meant to closely approximate reality. They are meant to be well-defined playable games where details (money, credit, the rate of interest, and so forth) are clearly defined and causality be considered. Yet he also claims that the models can demystify the lawful dynamics of market economies, such as perfect insight, rational expectations and the laws of Walras and Say.

Like most theoreticians in mainstream economics, Shubik is a very gifted mathematician and his analysis is staggeringly complex in these terms. However, the alleged symmetries between the outcomes of the playable games and those of general equilibrium theory are not emergent properties of a neural network of institutions that direct the future of a market economy in causal terms. They are simply artifacts of a mathematical analysis predicated on assumptions about the relationships between parts (economic actors and firms) and wholes (market systems) in general equilibrium theory. What is most important to realize here is that game theory, even in its most sophisticated forms, perpetuates belief in the real or actual existence of the invisible hand by disguising the natural laws of economics under a maze of different mathematical formalism. It does so by alleging that causal mechanisms in market economies are emergent properties of human decision-making within the lawful constraints of market processes as opposed to the transcendent god-like agencies that lurk behind the equations of general equilibrium theory.

Another fundamental problem faced by the game theorists is that since economic transactions in the real world are serial and multiple, any accurate depiction of the results of such transactions requires that games be repeated. However, repetition in non-cooperative games leads to the addition of more ad hoc assumptions about how each player will interpret the sequence of moves of other players. And this, as many game theorists have discovered, undermines the prospect that the outcome will be either fixed or determinant.

The work of the game theorists has proved very unsettling for many mainstream economists. In Walrasian general equilibrium theory, the natural laws of economics allegedly determine the optimal outcome of an economic process and economic actors are devoid of all distinctly human characteristics and obey fixed decision-making rules. In this theory, the realm of the economy is stable and unchanging and economic actors are viewed as supremely rational entities who do not talk back. In opening the box of human subjectivity, game theorists have been obliged to posit an increasing number of ad hoc variables to account for the decision-making of individual economic actors. This explains why the history of game theory is marked by a continual regression into the staggering complexities of language and culture.

Bargaining games led to cooperative games to non-cooperative games and to games where players are free to interpret the meaning of economic variables and the intentions of other players. More than half of a typical textbook on game theory is devoted to describing a staggering array of variants on particular games based on quite different conceptions of uncertainty and there is no sense of generality or unity. It now seems clear that the complexities of language and culture cannot be reduced to deterministic mathematical models and that attempts to do so have begun to undermine the validity of mechanistic rationality in general equilibrium theory. As economist Robert Sugden puts it:

There was a time, not long ago, when the foundations of rational-choice theory appeared firm, and when the job of the economic theorist seemed to be one of drawing out the often complex implications of a fairly simple and uncontroversial system of axioms. But it is increasingly becoming clear that these foundations are less secure than we thought, and that they need to be examined and perhaps rebuilt. Economic theorists may have to become as much philosophers as mathematicians.

Wassily Leontief, a Nobel Laureate in economics, has also expressed doubts about the efficacy of the orthodox neoclassical paradigm:

Page after page of professional journals are filled with mathematical formulas leading the reader from sets of more or less plausible but entirely arbitrary assumptions to precisely stated but irrelevant conclusions.Year after year economic theorists continue to produce scores of mathematical models to explore in great detail their formal properties; and the econometrics fit algebraic functions of all possible shapes to essentially the same sets of data without being able to advance, in any perceptible way, a systematic understanding of the structure and the operations of a real economic system.

Joseph Stiglitz.

The decision to award the 2001 Nobel Memorial Prize in Economic Science to economists who have done pioneering research on the imperfections of market systems is another indicator that mainstream economists have begun to question the validity of assumptions about the character of economic reality in the neoclassical economic paradigm. The winners, Joseph Stiglitz, George Akerlof, and Michael Spence, were chosen because they demonstrated that imperfect information in actual economic processes challenges the assumption that atomized economic actors are fully aware of complex economic variables in every transaction. According to these economists, these imperfections result in situations where the alleged lawful dynamics of closed market systems cannot allocate resources in the most efficient way and government must, therefore, intervene and make the necessary adjustments. For example, Stiglitz has shown that if the Securities and Exchange Commission did not enforce full disclosure in financial markets, investors would not have sufficient information to determine a proper value for stocks and some sectors of the market would have more information than others.

These criticisms and revisions of assumptions about the character of economic reality in neoclassical economic theory do not mean, however, that mainstream economists are in the process of developing a new theory predicated on a different set of assumptions. Virtually all of the most advanced theoretical work in mainstream economics is premised on the assumptions that market systems are, by varying degrees, closed, self-correcting, and self-sustaining and the primary impulse in these theories is to disclose the hidden dynamics that move market systems toward optimal states of equilibria with the use of increasingly more sophisticated mathematical techniques. For example, nonlinear analysis, particularly convex analysis, has been used to buttress the theoretical claims of the game theorists and the resulting mathematical formalism, as the work of Jeanne-Pierre Aubin illustrates, is staggeringly complex. But as the title of Aubins best-known book attests, Optima and Equilibria: An Introduction to Nonlinear Analysis. his attempt to uncover the immaterial logics which sustain the orderly workings of market systems is predicated on the assumption that these logics result from mechanisms associated with the operations of natural laws of economics.

More important, the vast majority of mainstream economists who work in business and government, including those at the International Monetary Fund and the World Bank, are not terribly concerned with the most advanced theoretical work in their discipline. Legions of these economists are engaged on a daily basis in developing analyses and making predictions that guide the decision-making of global economic planners and that serve to legitimate assumptions about economic reality in the neoclassical economic paradigm. Many of these planners are aware of the negative impacts of their decisions on the global environment and seek to minimize these impacts as long as profit margins can be maintained. These good intentions are, however, typically defeated by our now familiar culprit unscientific assumptions about economic reality that make it virtually impossible to resolve environmental problems in economic terms.

Because the predictions of neoclassical economic theory are not subject to empirical verification, the primary determinant of which theories are used to coordinate economic activities in particular market economies is the political process. Some theories may have more predictive value than others in dealing with specific sets of initial conditions. But the predictions themselves are merely useful approximations of tendencies to occur that point toward directions in which an economy may move under relative stable conditions. When the predictions are grossly inaccurate, as they almost invariably are under unstable market conditions, neoclassical economists typically attempt to explain, or explain away, the discrepancies by attributing the causes to market failures that can be potentially corrected or to events that occur outside the closed market system and interfere with its operation.

The Bank of Sweden Prize in Economic Sciences

Bank of Sweden Prize Medal.

The so-called Nobel Memorial Prize in economics was not among the five Nobel Prizes established by in his last will and testament in 1896. This prize, which is properly known the Bank of Sweden Prize in Economic Sciences in Memory of Alfred Nobel. was created and funded by the Central Bank of Sweden at the height of the Cold War in an effort to create the impression that one of the most politically charged issues in this war had been resolved. During the period in which the managers of this bank managed to convince the members of Nobel Prize committee to award this prize in the late 1960s, economists in both capitalist and communist countries claimed that their theories were scientific and that those used by economists on the other side of the ideological divide were not. In a situation where the competition between these very disparate conceptions of political and economic reality for global hegemony could have easily escalated into a full-scale nuclear war, the question of which theories were actually scientific was no trivial matter.

From the perspective of the bank managers, this question had already been answered because they had been taught in graduate school that the creators of neoclassical economic theory had transformed the study of economics into a rigorously scientific discipline like the physical sciences. Because they firmly believe that this was the case, the bank managers insisted that the new economics prize should be awarded, like the prizes in physics and chemistry, by the Swedish Academy of Sciences. The members of this academy initially resisted this proposal, and their principal reason for doing so was that scientists should not be involved in selecting the recipients of this prize because economics is a social science very different from the physical sciences. But after being persuaded that this arrangement might serve to curb the spread of communism, the scientists reluctantly accepted the proposal. However, this did not prevent the scientists from expressing their dissatisfaction in other ways, and one of them was to insist that the names of the economics laureates be engraved on the edge of their gold medals rather than on the more visible front of the gold medals awarded to the real Nobel laureates in physics and chemistry.