Testing Hedge Effectiveness Under SFAS 133
Post on: 4 Июль, 2015 No Comment

Testing Hedge Effectiveness Under SFAS 133
By John D. Finnerty and Dwight Grant
In Brief
How Effective is Highly Effective?
SFAS 133, Accounting for Derivative Instruments and Hedging Activities. marked a large step forward in FASBs quest to record financial instruments at fair value. The new accounting for hedges can introduce some complexity into the financial statements that can be avoided if the hedge qualifies as a highly effective hedge. Applying the definition of such a hedge, however, is subject to debate. The authors present three common methodologies for testing hedge effectivenessthe dollar-offset method, the variability-reduction method, and the regression methodand analyze them. They recommend against using the dollar-offset method, which is more sensitive to small changes, but also stress the importance of examining all the specifics of the situation.
SFAS 133, Accounting for Derivative Instruments and Hedging Activities. was issued because the effects of the increasing quantity and variety of derivatives used by companies were not transparent in the financial statements. SFAS 133 standardizes the accounting treatment for derivative instruments by requiring all entities to report derivatives as assets and liabilities on the balance sheet at their fair value. This change is a step toward FASBs objective of having all financial instruments measured and reported at fair value.
SFAS 133 recognizes three categories of hedges: fair value, cash flow, and foreign currency. A fair value hedge offsets the price risk of a recognized asset or liability or an unrecognized firm commitment. A cash flow hedge offsets the variability of the cash flow of a balance sheet item or a forecast transaction. SFAS 133 describes foreign currency hedges separately, even though the majority are fair value or cash flow hedges, in order to preserve the hedging concepts embedded in SFAS 52.
If a derivative qualifies as a highly effective hedge, SFAS 133 permits companies to match the timing of the gains and losses of hedged items and their hedging derivatives. For a fair value hedge, SFAS 133 permits the hedger to record the change in the fair value of the hedged item concurrently with the gain or loss on the hedging derivative. For a cash flow hedge, the effective portion of any changes in the hedging derivatives fair value is recorded in other comprehensive income until the change in the value of the hedged item is recognized in earnings. If a derivative does not qualify as a hedge, changes in its value must be reported in quarterly earnings.
In principle, a hedge is highly effective if the changes in fair value or cash flow of the hedged item and the hedging derivative offset each other. To permit as precise matching as possible, the hedged item can be any portion of a balance sheet item, firm commitment, or forecast transaction; the hedge position can be any portion of a derivative. Similarly, the hedger can exclude from the hedge calculations anticipated changes in value that are not part of the risk being hedged. For example, if a derivative is priced at a discount to the current spot price and is expected to appreciate toward the current spot price as the derivative approaches expiration, the company can account separately for that expected appreciation.
To qualify a derivative position for hedge accounting, the hedging entity must specify the hedged item, identify the hedging strategy and the derivative, and document by statistical or other means the basis for expecting the hedge to be highly effective in offsetting the designated risk exposure. The documentation step is called prospective testing, and it must be done before entering into the hedge, and on an ongoing basis, to justify continuing hedge accounting. The hedger must also regularly perform retrospective testing to determine how effective the hedging relationship has actually been. Unless a specific exception applies, Section 2 of Appendix A of SFAS 133 requires the use of statistical or other numerical tests to demonstrate that a hedge is highly effective and thus qualifies for hedge accounting under SFAS 133 [for details on exceptions, see guidance provided by FASBs Derivatives Implementation Group (DIG)]. SFAS 133 does not endorse any specific testing methodology. The hedger must select the methodology, choose the measurement period and observation frequency, and specify an appropriate test statistic.
Defining and testing a measure of hedge effectiveness represents an important and potentially challenging aspect of hedge accounting. Failure to meet the challenges hedge accounting presents may introduce substantial volatility into reported earnings.
Defining Highly Effective
What, exactly, is a highly effective hedge? One response is that a highly effective hedge substantially offsets the change in the fair value (or the cash flow) of the hedged item. That is, if the hedged item in a fair value hedge appreciates by $100,000, then there is some range of decline in values of the hedge that can be defined as substantially offsetting this change. Defining this range is a matter of subjective judgment. A highly effective hedge has been suggested as offsetting at least 80% of this change and no more than 125%. Then the acceptable range of the change in value for the derivative would be between $80,000 and $125,000. This method of testing for effectiveness has the additional merit that it leads directly to the accounting treatment of the change in value of the derivative. To the extent that the sum of the changes in values is not zero, there is an element of ineffectiveness in the hedge that is included in current income. Thus, even when a hedge is determined to be highly effective, there is an impact on current earnings when there is not an exact offset of the hedged risk. If, for example, the change in value of the derivative were $110,000, then the hedge would be highly effective, because this change in value falls within the specified range and hedge accounting would report an effect on income of +$100,000 $110,000 = $10,000. This idea of offsetting has found its way into the hedge effectiveness testing literature in the form of the dollar-offset method of testing, discussed in detail below.
A second response to this question is that a highly effective hedge substantially offsets risk associated with the change in the fair value (or the cash flow) of the hedged item. A widely accepted measure of risk is variance. Estimating variances requires multiple observations. To extend the example above, suppose that over four quarters the changes in fair value of the hedged item were +$100,000, +$40,000, $120,000, +$5,000, and the corresponding changes in the fair value of the derivative were $110,000, $35,000, + $128,000, $8,000. The recorded hedged income effects would then be 10,000, +$5,000, + $5,000, $3,000. The variance without hedging is 8.623 billion (dollars squared) and the variance of the income stream with hedging is 0.07 billion (dollars squared). Hedging eliminated 99% of all of the variance of income. This variance measure of effectiveness formalizes the clear conclusion that the hedge eliminated most of the risk.
Methods of Testing Hedge Effectiveness
There are three primary methods of testing the hedging effectiveness of forwards, futures, and swaps when the critical terms of the hedging derivative and the hedged item are not identical: the dollar-offset method, the variability-reduction method, and the regression method. The following examples illustrate each method. More detailed calculations of each method can be seen in the example described below and illustrated in Exhibit 1.
Dollar-Offset Method
The dollar-offset method, which has some historical significance for the accounting profession (DIG Issue E7), compares the changes in the fair value or cash flow of the hedged item and the derivative. The dollar-offset method can be applied either period by period or cumulatively (DIG Issue E8). For a perfect hedge, the change in the value of the derivative exactly offsets the change in the value of the hedged item. Therefore, the ratio of the cumulative sum of the periodic changes in the value of the derivative and the cumulative sum of the periodic changes in the value of the hedged item would equal one in a perfect hedge (after multiplying the ratio by negative one to adjust for the two sums having opposite signs in a hedging relationship).
Of course, perfection is not necessary to qualify for hedge accounting. In a speech at the SECs 1995 Annual Accounting Conference, a member of the SECs Office of the Chief Accountant articulated an 80/125 standard for hedge effectiveness as measured by the dollar-offset method. This became a guideline for assessing the hedge effectiveness of futures contracts under SFAS 80, and has carried over to SFAS 133.
Anyone choosing this test should be aware that researchers question its reliability because of its excessive sensitivity to small changes in the value of the hedged item or the derivative. For example, suppose that the hedged item is inventory valued at $1 million and the hedge is a short position in a futures contract. At the end of the quarter, suppose that the value of the inventory increased by some small amount, say $10,000 (1%). The short futures position will decrease in value by $10,000, offsetting the change in the value of the underlying asset, plus or minus the change in the futures basis. If the change in the basis is as little as 0.33% of the notional value ($3,333), then the dollar-offset method implies that the hedge is ineffective because the short futures value change is 33% of the inventorys value change. Canabarro (1999) has shown that under reasonable assumptions about the distribution of changes in prices, the 80/125 standard rejects as ineffective 36% of all hedges when the coefficient of determination (correlation squared) R2 is 0.98.
Variability-Reduction Method
The variability-reduction method and the regression method are closely related. The difference is that the variability-reduction method assumes that the risk-minimizing derivative position is equal and opposite to the hedged item, that is, a one-to-one hedge. The regression method assumes that a more effective hedge is based on a statistical estimate of the risk-minimizing hedge.
If a one-to-one hedge performs perfectly, the change in the value of the derivative exactly offsets the change in the value of the hedged item. The variability-reduction method compares the variability of the fair value or cash flow of the hedged (combined) position to the variability of the fair value or cash flow of the hedged item alone. This method places greater weight on larger deviations than on smaller ones by using the squared changes in value to measure ineffectiveness. The preferred test statistic for this method is the proportion of the hedged items mean-squared deviation from zero that the hedge eliminates. To calculate the test statistic, subtract from one the ratio of the sum of the squared periodic changes in the hedge and the hedged item to the sum of the squared changes in the hedged item.
The mean-squared deviation from zero is used because the variance ignores certain types of ineffectiveness. For example, suppose that the change in the value of the hedged position is always $0.20. If this is used in the numerator, the test statistic is 1.0 because the variance measures the variability around the mean of $0.20. However, because this variance is $0.20 in every period in this example, the offset is not perfect. By using mean-squared deviations, the test statistic reflects the lack of offset in the means.
The critical value for determining how large a reduction in variability is sufficient to demonstrate hedge effectiveness must be specified in order for this measure to be useful. Because of the similarity of this test to the regression method test, the authors believe that a standard of 80% is appropriate.
Regression Method
The prospective measure of hedging effectiveness is based on the adjusted R2 produced by a regression in which the change in the value of the hedged item is the dependent variable and the change in the value of the derivative is the independent variable.
Ederington (1979) showed that the estimated slope coefficient is the variance-minimizing hedge ratio. Given the definitions of X and Y, the slope of this regression equation should be negative and close to 1.0. In terms of a prospective effectiveness test, if the adjusted R2 is greater than 80%, then a hedge ratio equal to the regression slope coefficient would have been highly effective. The interpretation of the intercept term is also important. It is the amount per (data measurement) period, on average, by which the change in value of the hedged item differs from the change in value of the derivative. Because the hedge should aim for a combined change in value of 0.0, the hedger should account separately for the intercept term.
The retrospective test of effectiveness recommended for the regression method is essentially the same as the test for the variability-reduction method. It differs in that it explicitly allows for a hedge ratio that differs from 1.0 and for the exclusion of part of the change in the value of the derivative; that is, that the hedger implements a hedge based on the results of the regression estimates. The retrospective regression variability reduction test statistic is 1 A / B, where A is the sum of the squared differences between the estimated hedge value using the regression intercept and coefficient and the hedged item, and B is the sum of the hedged items values squared.
In principle, the hedger can use the regression coefficients or any other values that will make the hedge more effective. In practice, the regression intercept and coefficient may be sufficiently close to 0 and 1 that the hedger implements a simple one-to-one hedge. In that case, the variability-reduction method and the regression method would yield identical retrospective measures of effectiveness.
Implementing Hedge Effectiveness Testing
The hedger must identify the hedged item and the derivative, an objective, the data and time period to be used, and a test method with a standard for highly effective. SFAS 133 provides some guidance on testing. Retrospective testing and the update of prospective testing should be performed at least each quarter or each time a financial statement or earnings are reported until the hedge is unwound (SFAS 133 and DIG Issue E7). Data used in retrospective testing must include the actual results since the inception of the hedge and may include additional historical data. Tests used to document hedge effectiveness must be consistent with the hedgers stated approach to risk management, and the hedger must generally use the same method to test the effectiveness of similar hedges, unless different methods are explicitly justified.
SFAS 133 provides flexibility with respect to the frequency and duration of data observation used in the effectiveness testing. Some commentators have argued that the testing interval should match the hedges time horizonfor example, using annual data to evaluate whether a particular derivative is an effective hedge of a 12-month exposure. Matching exposure and measurement periods, however, may limit the number of independent observations available for statistical testing. This may not be feasible given available data, or it may not be appropriate because the market changed substantially over that period of time. Therefore, the authors suggest using more frequent observations of the data over a shorter historical time period.
As indicated above, because SFAS 133 does not specify a bright-line test to distinguish highly effective hedges from less effective or ineffective hedges, the interpretation of highly effective is a matter of judgment. SFAS 133 does say that the high-effectiveness requirement is intended to have the same meaning as the high correlation requirement of SFAS 80. With regard to the dollar-offset method, this requirement has been interpreted to mean that the cumulative changes in the hedging derivative should offset between 80% and 125% of the cumulative changes in the fair value or cash flows of the hedged item. For the regression method, it has been interpreted to mean that the regression of changes in the hedged item on changes in the derivative should have an adjusted R2 of at least 80%.
Analysis
To illustrate the methods described above, consider the case of a U.S. company that is considering, on December 31, 1999, hedging a purchase of aluminum that the company expects to make on December 31, 2000. The derivative is a long forward contract on the London Metals Exchange (LME). On December 31, 1999, the cash price for delivery to the companys plant is $1,712.30 per metric ton, compared to the cash and one-year forward prices on the LME of $1,630.50 and $1,641.00, respectively.
To determine whether the one-year forward contact is expected to provide a highly effective hedge, the company collected 1998 and 1999 monthly cash prices for delivery of aluminum to its plant and calculated forward prices on the LME. The example illustrates the companys prospective test of hedging effectiveness as of December 31, 1999, and its subsequent retrospective tests of effectiveness for the four quarters of 2000. For the dollar-offset method, the ratio is calculated for each month and each quarter. For the variability-reduction and regression method, all calculations for each quarter are made using the most recent two years of data.
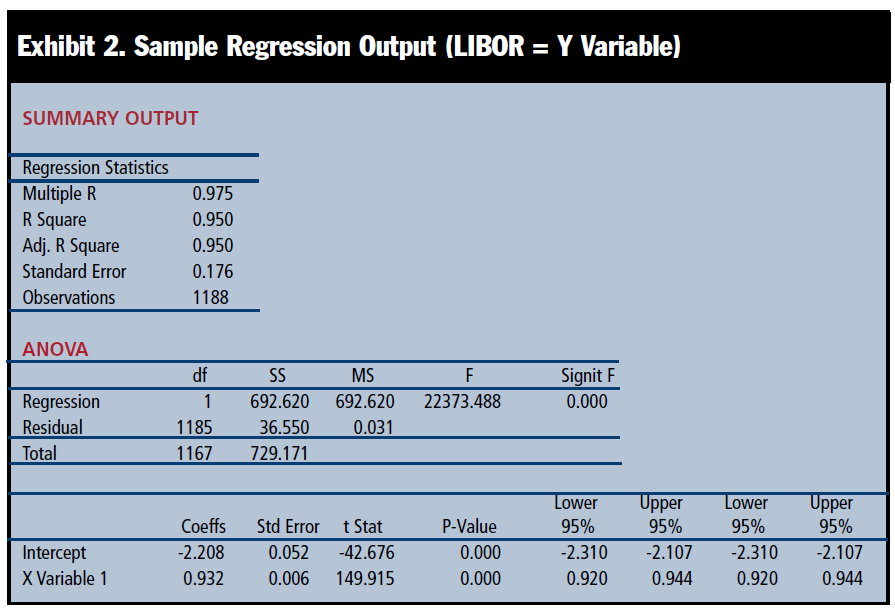
Exhibit 1 illustrates the calculations using the quarterly data. (Excel spreadsheets for this and all other calculations are available from www.cpajournal.com .) Columns 3 and 4 contain the prices of the derivative, and column 5 includes the prices of the hedged item. Columns 6 and 7 compute the changes in the values of the derivative and the hedged item. Column 8 calculates the dollar-offset ratio. Note that on a quarterly basis the ratio falls outside the acceptable range two of the eight quarters in the prospective period but none of the quarters in 2000. Column 10 is the square of the changes in the values of the hedged item, and column 11 is the square of the changes in the hedged position. Column 12 computes the squares of the hedged position based on the regression estimates shown in Exhibit 2.
Columns 10 and 11 are used for the variability-reduction method. The prospective test is based on the sum of the first eight items in each column:
1 2, 658.36 = 0.97
The retrospective test for the first quarter of 2000 is calculated in the same way, using the sums of quarters 2 to 9. The complete set of calculations for all quarters are in the spreadsheets. Columns 10 and 12 are used for the regression method. The prospective test is based on the adjusted R2 of the regression, 0.99. The retrospective test for the first quarter of 2000 is calculated using the values of the intercept and coefficient estimated for the eight quarters in 1998 and 1999 and implemented in the first quarter of 2000.
1 724.08 = .99
Exhibit 3 reports the test of effectiveness for the variability-reduction method and the regression method for all four quarters of 2000, using quarterly and monthly observations. The results are quite similar. The regression method records slightly higher levels of variability reduction because the estimates of the intercept and the slope are quite stable over time and, therefore, using them enhances the results relative to the values of 0 and 1.0 that are implicit in the variability-reduction method. The results using quarterly data indicate that the derivative eliminates virtually all of the variability of the hedged item, while the results using the monthly data indicate a variability reduction around 90%.
Recommendation
The dollar-offset method is well established with the articulated 80/125 standard for effectiveness. Companies adopting this method should be aware that the test statistic is sensitive to observations with small changes in value. Because of this, the dollar-offset method identifies a relatively high percentage of hedges as not highly effective, even when the variability reduction approaches 98%. The authors believe this is a serious flaw. The variability-reduction method and the regression method both measure effectiveness in terms of risk reduction. When the derivative is very similar to the hedged item, it appears likely that the differences between these two methods will be small. If the derivative and the hedged item are not very similar, the regression method will be superior, if the variance-minimizing hedge ratio deviates materially from 1 and is stable over time.
While the authors believe the examples above are a good guide for practice, the company and its auditor must concur on the appropriate process for testing hedge effectiveness. A number of important and more complex issues, including dynamic hedging, option hedging, and the use of multiple derivatives to hedge, may also need to be addressed, depending upon the specific situation.
John D. Finnerty is a principal with Analysis Group/Economics and a professor of finance at Fordham University, New York City.
Dwight Grant, PhD. is the Douglas M. Brown Professor of Finance at the University of New Mexico.














