Price and Yield Calculations

Price and yield are both very important when it comes to determining the value of U.S. Treasury securities.

U.S. Treasury Notes and Bonds are traded according to what is called the “quoted price” (also known at the “clean price” or “quote”). For a better understanding of price quoting conventions and calculations for Notes and Bonds, refer to the page titled “Invoice Price.”

Though price is important to consider, it is not especially useful when evaluating the relative value of Treasury securities. There are hundreds of Treasury issues outstanding, all with different coupons and maturities, trading at a variety of prices. Though many of these issues were auctioned at about “100” (100% price is also called “par”), fluctuating interest rate levels and shifting markets mean that many have traded up or down in the secondary markets.

It seems intuitive that Treasuries with higher coupons should be worth more (and thus traded at higher prices) than Treasuries of similar maturities with lower coupons. But to what degree should the value of the security reflect the coupon?

That’s where yield comes in. Yield (also called “Yield to Maturity” or “YTM”) is the effective rate of return (or internal rate of return). In other words, yield is interest rate of the Treasury security, taking into account price and underlying cash flows until maturity.

Notes and Bonds have known cash flows: coupons are paid every six months, and the principal is paid at maturity. One can learn the price of a Note or Bond by calling an institutional salesperson or broker or by looking at market data screens. Of course, prices can also be negotiated when two parties are considering a trade.

With all these known factors (price, coupon, and principal), one can mathematically calculate the yield on a security. Yield is critical when assessing relative worth; all else being equal (risk, maturity, etc.), most investors will choose those securities with higher yields.

Yield to Price

The opposite is also true. If you know what yield you’re aiming for, you can calculate the price at which you should purchase the security.

Basic Finance Math

Before we get into Price/Yield Calculations, some review of basic finance math ( “Time Value of Money” calculations) will be helpful. Those familiar with this topic may skip this section.

Terminology

• PV – Present Value
• R – Interest Rate
• N –Number of Years
• F – Compounding Frequency (1 – annual, 2 – semi-annual, 4 – quarterly)
• FV – Future Value
• Mathematical notation. “*” multiplication, “/” division, “^” exponent (raised to the power)

The general formula for the Time Value of Money is:

FV = PV * (1 + R / F) ^ (N * F)

Try this example: What is the Future Value of $1,000 invested for one year assuming a 5% interest rate, compounded annually?

In this example, PV = $1,000, R= 0.05 (5% as a decimal), N = 1, and F=1. Plugging this in to the equation we get:

FV = $1,000 * (1 + 0.05 / 1) ^ (1 * 1) = $1,050

Now use the same example, but assume interest is compounded semi-annually (F = 2). We get:

FV = $1,000 * (1 + 0.05 / 2) ^ (1 *2) = $1,050.625

PV in Terms of the Other Four Variables

When investors are evaluating a cash flow in the future, they often use the following version of the Time Value of Money formula:

PV = FV / [ (1 + R/F) ^ (N * F) ]

This equation essentially states that we can calculate the Present Value (PV) of a future cash flow if we know its Future Value (FV). the Interest Rate (R), its Compounding Frequency (F) and the Number of Years (N) over which it is earning interest.

U.S. Treasury Note and Bond Cash Flows

The future cash flows of U.S. Treasury Notes and Bonds are all known. Coupon payments are made semi-annually, (on the anniversary and “half-anniversary” of the maturity date). Specifically, Treasury Notes pay coupons on the 15 th or at the end of the month; T-Bonds pay interest on the 15 th .

The principal or “corpus” is paid back at maturity. There are no other cash flows for Notes and Bonds.

PV in Bond Speak

Starting with the Present Value (FV) formula above, substituting 2 for Frequency (because Notes and Bonds pay coupons semi-annually), using “Y” instead of “R” to represent the Interest Rate (because in Wall Street speak a rate of return is called a yield), and reformatting a little we get:

PV = FV / (1 + Y/2)

In finance this is sometimes called “discounted cash flow.” The Future Value of a cash flow is discounted to Present Value (because it is worth less today than it will be in the future because of the opportunity cost of money).

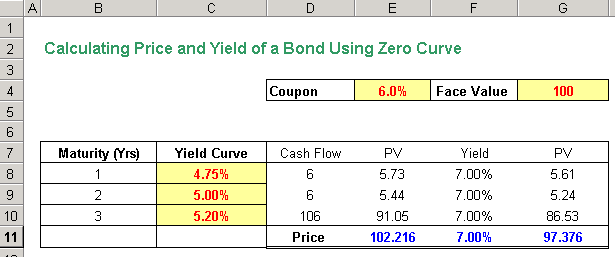
Price/Yield Calculation

As described before, all the cash flows of a Note or Bond are known. Therefore, we can calculate the present value of each individual cash flow using this formula. And by adding all the Present Values of all the individual cash flows we arrive at the Present Value of the entire Note itself, which is the Price of the Note.

Price is the invoice price, which is equal to the quoted price plus accrued interest. (Refer to the article on “Invoice Price” if you’d like a to learn more about these concepts).

We must divide the coupon by 2 because it is quoted in annual terms but paid semi-annually—each payment is really half the coupon rate.

The equation has two unknown variables: price and yield. If we know one variable, we can calculate the other. So if an investor has a particular yield in mind, he can plug it into the formula and decide whether he is willing to pay the price it generates.  Conversely, if he receives a price quote from a screen or dealer, the investor can compute the yield. And by comparing this yield with the yields of other securities, he can determine whether the price seems suitable.

Notice that, in this formula, if the value of “Y” increases, each cash flow decreases because the denominator gets larger while the  numerator remains unchanged (making the ratio smaller). Thus the sum of the individual cash flows is also smaller. Generally speaking,  when yield goes up, price falls, and when yield falls, price rises.

Price to Yield

The Yield to Price calculation is straightforward because we can represent price as a function of yield (P = F(Y)), as illustrated above. The inverse calculation is a bit trickier, because there is no simple equation for representing yield as a function of price (Y= F(P)).

The calculation requires some understanding of calculus, particularly a tool of numerical analysis called the Newton-Raphson iteration method (created by Isaac Newton and Joseph Raphson). This is beyond the scope of this article, but an introductory calculus textbook might provide some interesting explanations for those of you are mathematically inclined.

If you don’t like math, not to worry: price/yield calculations are widely used and readily available from Bloomberg and Reuters workstations, most trading systems, Microsoft Excel, and other resources.

As usual, the best way to understand these concepts is to work through a real example. We’ll use the following 2-Year Treasury Note:

• Issue Date: July 31, 2011
• Coupon: 0.375%
• Maturity: July 31, 2013

On August 4, 2011 (Trade Date), this Note was trading at “100-07” for settlement on August 5, 2011 (Settlement Date, next business day).

Cash Flows of the 2-Year Note

This U.S. Treasury Note has four Coupon payments: January 31, 2012, July 31, 2012, January 31, 2013, and July 31, 2013 (the maturity date, on which 100% of the principal is also due). Recall that each coupon payment is actually half the annual coupon rate. So in our example, each coupon is 0.1875 (0.375/2).

Thus the future cash flows for this Note are:

• 01/31/2012   0.1875% Coupon
• 07/31/2012   0.1875% Coupon
• 01/31/2013   0.1875% Coupon
• 07/31/2013 100.1875% Coupon + 100% Principal

Invoice Price

In this example, we’re buying the Note on August 4, 2011 for settlement on August 5, 2011. So in addition to paying the quoted price of “100-07” on August 5, 2011, we have to add accrued interest (refer to article on “Invoice
 Price”).

Invoice Price = Quoted Price + Accrued Interest

Intermediary Calculations

• Quoted Price = 100 + 7/32 = 100.21875
• Accrued Interest = (Coupon / 2) * (Days interest accrues) / (Days in Coupon Period)
• Days on which interest accrues (July 31, 2011 up to and including August 5, 2011) = 6 days
• Days in Coupon Period (July 31, 2011 to January 31, 2012) = 184 days
• Accrued Interest = (0.375/2) * (6/184) = 0.00611413

Invoice Price (or Price) = 100.21875 + 0.00611413 = 100.2248641

Fractional Period

August 5, 2011, the settlement date, differs from the semi-annual anniversary dates of the 2-Year Note. Thus there is a fractional period to “discount” the cash flows. In our example, the next coupon payment will be made on January 31, 2012, which is 178 days from the August 5, 2011 settlement date (184 days minus 6 days from the July 31, 2011 issue date).

The next coupon on January 31, 2011 is not “1” period away, but instead 178/184 of a period away, which is equal to 0.967391304 (we’ll call this number “T”). Not only the next coupon date but all other future cash flows must take into account this fractional period.

Putting it All Together

Using our 2-Year Note example and plugging into the Price/Yield formula, we get:

where T is a constant that was previously calculated above.

Now we have all the variables filled in except for one unknown, Y. Solving for Y (using Excel or a bond calculator), we get:

Yield  =  0.264% based on the Quoted Price of “100-07”

The math is quite cumbersome; thank goodness for computers and software.

Price Yield Curve

If you were to calculate the prices for a range of yields and plot them on axes, you’d have generated a Price-Yield curve.

Price-Yield Curve

Price and yield are used constantly by market participants—Wall Street trades securities on price, but yield is used to determine value. Since the relationship between price and yield is so widely used, you don’t have to perform the calculations yourself; there are many tools that can do them for you. Still, and understanding of some of the underlying math, conventions, and financial concepts can be both interesting and useful.