Loading.

PPT Bond Portfolio Management Strategies: Basics II PowerPoint presentation | free to download

Bond Portfolio Management Strategies: Basics II

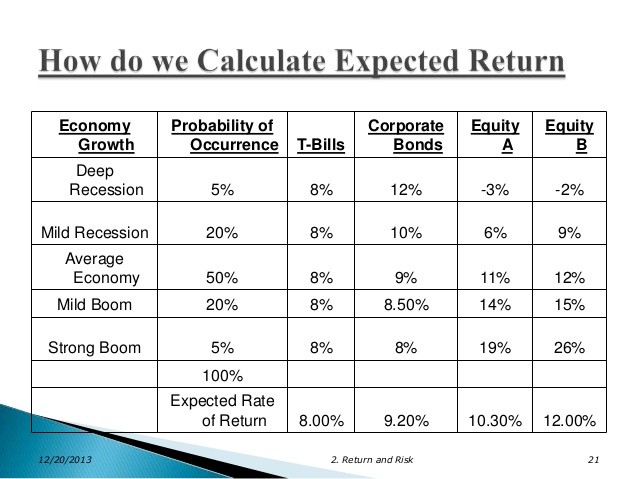
What are theoretical spot rates and forward rates and how do we compute them. However, price changes are not linear, but a curvilinear (convex) function. 15. PowerPoint PPT presentation

Bond Portfolio Management Strategies

• What is the convexity for a bond, how do you

estimating a bonds price volatility?

Theoretical spot rates

• We have seen that using STRIPS we can determine

the spot rate for a particular maturity.

slightly different from those observed in STRIPS

because the stripped securities are not as liquid

as the current Treasury issues.

Theoretical spot rates

• We can compute a set of theoretical spot rates

through a process referred to as boot-strapping.

of a package of zero coupon securities that

duplicates the coupon bonds cash flows.

Forward rates

• Forward rates represent the markets expectation

of future short-term rates.

T-bills, we can extrapolate this forward rate.

Interest Rate Sensitivity

• Interest rate sensitivity is the amount of bond

price change for a given change in yield.

Trading strategies based on interest rate

sensitivity

• If you expect a decline (increase) in interest

rates, you want a portfolio of bonds with maximum

(minimum) interest rate sensitivity.

Macaulay Duration Measure

• The Macaulay Duration can be calculated as
• Where
• t time period in which the coupon or principal

payment occurs

Determining interest rate sensitivity

• An adjustment of Macaulay duration called

modified duration can be used to approximate the

bond price change to changes in yield.

Trading Strategies Using Modified Duration

• Longest-duration security provides the maximum

Bond Convexity

• Modified duration is a linear approximation of

bond price change for small changes in market

yields

Determinants of Convexity

• The convexity is the measure of the curvature and

can be calculated as

The change in price due to convexity is then

Determinants of Convexity

• There exists a(n)
• Inverse relationship between coupon and convexity
• Direct relationship between maturity and

convexity

Modified Duration-Convexity Effects

• Changes in a bonds price resulting from a change

in yield are due to

the characteristics of the bond (its convexity)

and the size of the yield change

Limitations of Macaulay and Modified Duration

• Percentage change estimates using modified

duration only are good for small-yield changes.