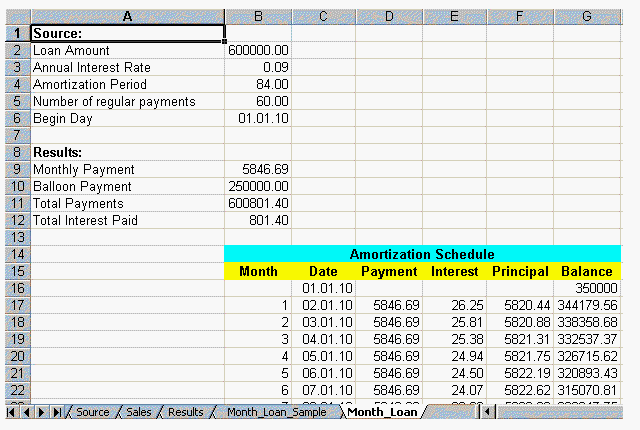
by Landon M. Scott on May 21, 2011

Remember that the Internal Rate of Return (IRR) is not a unique equation but is instead a process of iteration of the discount rate in the calculation of Net Present Value (NPV).  It is adjusting the discount rate in the calculation of NPV until a zero NPV is found; the discount rate of a series of cash flows that yields a zero NPV is the Internal Rate of Return (IRR).

For a real estate deal, for example, that is 100% equity and not leveraged with debt, the cash flows involved are usually a negative purchase price, followed by the Net Operating Income for a period of years, followed by the final year’s Net Operating Income plus the net sales value of the property. If you run an IRR calculation on that series of cash flows, you will get the discount rate that would yield a zero NPV – the Internal Rate of Return.

For a real estate deal that utilizes financing and is therefore “leveraged” with debt, the cash flows involved are the negative equity paid at purchase (so if the loan was 70% Loan to Value on a $100,000 apartment, the first cash flow would be -$30,000), followed by the Before Tax Cash Flows for each year (BTCF is NOI less debt service), followed by the final year’s BTCF plus the net sales value of the property (which in this case would be net of the balance of the loan, unlike in the unleveraged IRR above). This “Leveraged IRR” is typically greater than the “Unleveraged IRR”, all else equal.

An assumption in the IRR calculation is that the annual cash flows are reinvested at a rate equal to the Internal Rate of Return. So if you stash your Net Operating Income in a savings account that yields 3% but IRR tells you the rate or return on your apartment is 10%, your real return will be less than 10%. This isn’t, as so many people seem to believe, a machination by some slick mathematician, but rather a function of the fact that discounting (the math used to translate future cash flows into a Present Value) is simply the opposite of compounding; instead of compounding a number by the same percentage out in the future, we are discounting Future Values back to today by the same percentage. It’s just the math involved.

In NPV calculations, one of the inputs is the cost of capital (aka the discount rate). You discount everything in the future by the cost of capital (because it is what you are giving up by not placing that money in an alternate investment which would otherwise have enjoyed compounding returns). IRR calculations just tell you what the implicit cost of capital is (because an investment that yields exactly your cost of capital will yield a zero NPV, the reason why you should accept deals that give you a positive NPV and reject those that yield a negative NPV).

If you don’t have alternate investments where you can park your free cash flows at or above the IRR of a specific deal, you can calculate a more realistic return by using the Modified Internal Rate of Return (MIRR).

I will post a follow up to this titled Leveraged IRR in the near future with examples.

Comments on this entry are closed.