This article builds on the basic financial principles from Comparing Investments to show how bond prices are calculated. It does not discuss why prices change, nor should it be used to predict future performance. Please consult the forum for guidance.

Microsoft Excel was used for this article. However, Open Office Calc will work equally well and is a free application that is supported on several operating systems. Open Office financial functions are very similar to Microsoft’s functions. [1]

Excel formulas are shown where possible. The figures were created from the examples. All examples are available for download at the bottom of this page.

Contents

Required yield

The interest rate, or discount rate. that the investor wants from investing in a bond is called the required yield. Required yield is often specified as an annual interest rate. For purposes of calculating cash flows. a bond’s semiannual interest rate is conventionally set to one-half the annual interest rate.

However, note that using a periodic rate as one-half the annual interest rate will result in an effective annual yield that is greater than the annual interest rate (an error in the actual interest rate). [2]

Pricing a bond

Bond price is the sum of the present value of the cash flows, which is determined by adding: [3]

1. The present value of the (semiannual) coupon payments
2. The present value of the par or maturity value

This is shown mathematically as:

where p = Price c = Semiannual coupon payment i = Periodic interest rate (required yield/2) n = Number of periods (number of years x 2) M = Maturity value

As the semiannual coupon payments are equivalent to an ordinary annuity. the same present value formula used for Comparing Investments applies.

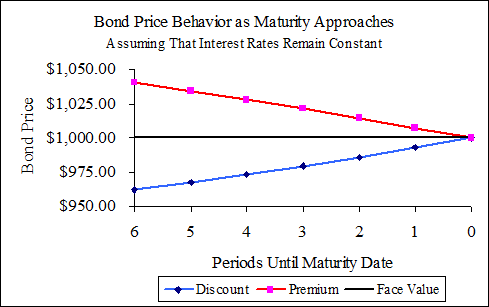
Price / Yield curve

1. PV = Bond price. A sum of the cash flows.
2. FV = Par (Maturity) value. Set by the bond issuer, cannot change.
3. PMT = Coupon rate. The interest rate set by the bond issuer, cannot change.
4. N = Number of compounding periods, typically twice a year (semiannual). Set by the bond issuer as the Maturity date, cannot change.
5. I = Required Yield, an interest rate desired by the investor. Normally specified as an annual rate (Semiannual rate = Required Yield / 2).

• Type = 0. Ordinary annuity. Does not change.

Since I is in the denominator of the bond price equation, increasing I will decrease the price (the other variables are fixed). Therefore, a bond’s price will always theoretically move in the opposite direction to its yield.

As yields change in the marketplace, the only variable that can compensate for these changes is price (sum of PV). [4] This why bond charts show price and yield- they are the only 2 variables that are not set by the bond. Change one and the other follows.

Bond Price/Yield Relationship

Notice how the price of a bond follows the basic property of present value — as the interest rate rises, the price drops and vice versa. [5] The curve of this graph has a convex shape, which can have significant implications for the investment properties of a bond. [6]

Coupon rate, required yield, and price relationships